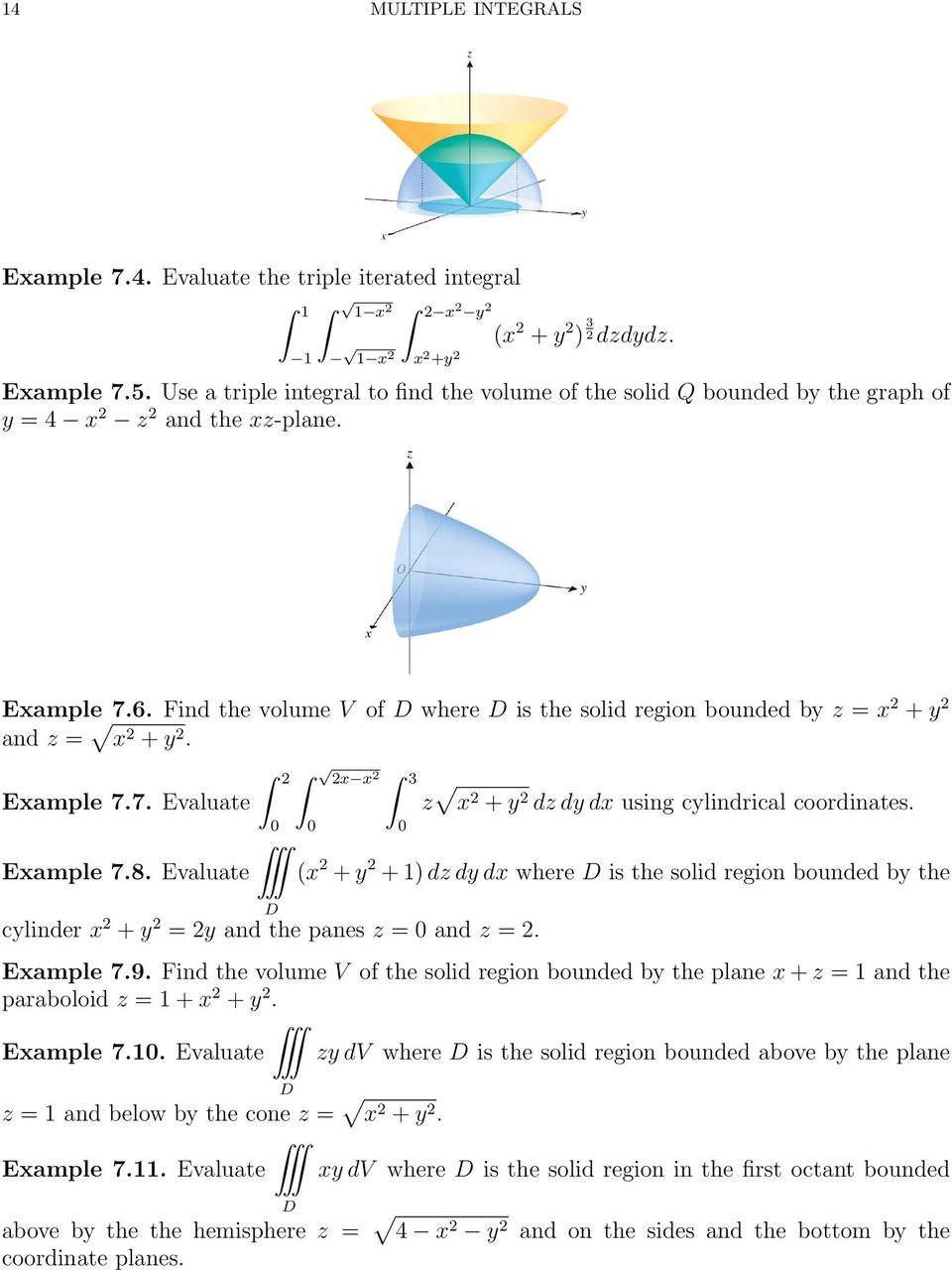
(a) Find and identify the traces of the quadric surface −x 2 − y 2 z 2 = 1 and explain why the graph looks like the graph of the hyperboloid of two sheets in Table 1 (b) If the equation in part (a) is changed to x 2 − y 2 − z 2 = 1, what happens to the graph?Sketch the new graphFree PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystep(a) Find and identify the traces of the quadric surface $ x^2 y^2 z^2 = 1 $ and explain why the graph looks like the graph of the hyperboloid of two sheets in Table 1 (b) If the equation in part (a) is changed to $ x^2 y^2 z^2 = 1 $, what happens to the graph?
How To Plot Coordinates c Bitesize
Plot x^2+y^2+z^2=1 in matlab
Plot x^2+y^2+z^2=1 in matlab-The projected region R in the x−y plane is x2 y2 = 4 Where the two surfaces intersect z = x2 y2 = 8 − x2 − y2 So, 2x2 2y2 = 8 or x2 y2 = 4 = z, this is the curve at the intersection of the two surfaces Therefore, the boundary of projected region R in the x − y plane is given by the circle x2 y2 = 4 So R can be treated as aXz plane traces create ellipses This tells us the surface is an ellipsoid because there are 2 ellipsoid graphs to choose from, we look at the major axis in the function and pick the graph with the corresponding major axis x axis radius = 1, y axis radius = (sqrt (1/4))^2 z axis radius = (sqrt (1/9))^2 We see the major axis is the x axis, and the corresponding graph is VII


Answer In Calculus For Ero
3dprinting, solidworks f(0,0,0) is 0, not 1 (the isosurface level), so you only get points drawn completing the cones if there are enough points near the origin that happen to have value 1 But when you switch to linspace(,,), the closest coordinates to the origin are at about 105, leaving a gap of about 21Macias (ygm97) – Homework 13 – staron – () 17 Consequently, volume = 2 π 1 − 5 e 4 cu ft/hour 026 100 points The solid shown in lies below the graph of z = f (x, y) = 3 x 2 − y 2 above the disk x 2 y 2 ≤ 1 in the xyplane Determine the volume of this solid 1Let {eq}f(x,y,z)=x^2y^2z^2 {/eq} and let S be the level surface defined by f(x,y,z) = 4 {/eq} lies on a portion of the surface that is the graph of a function Solve for z as a function
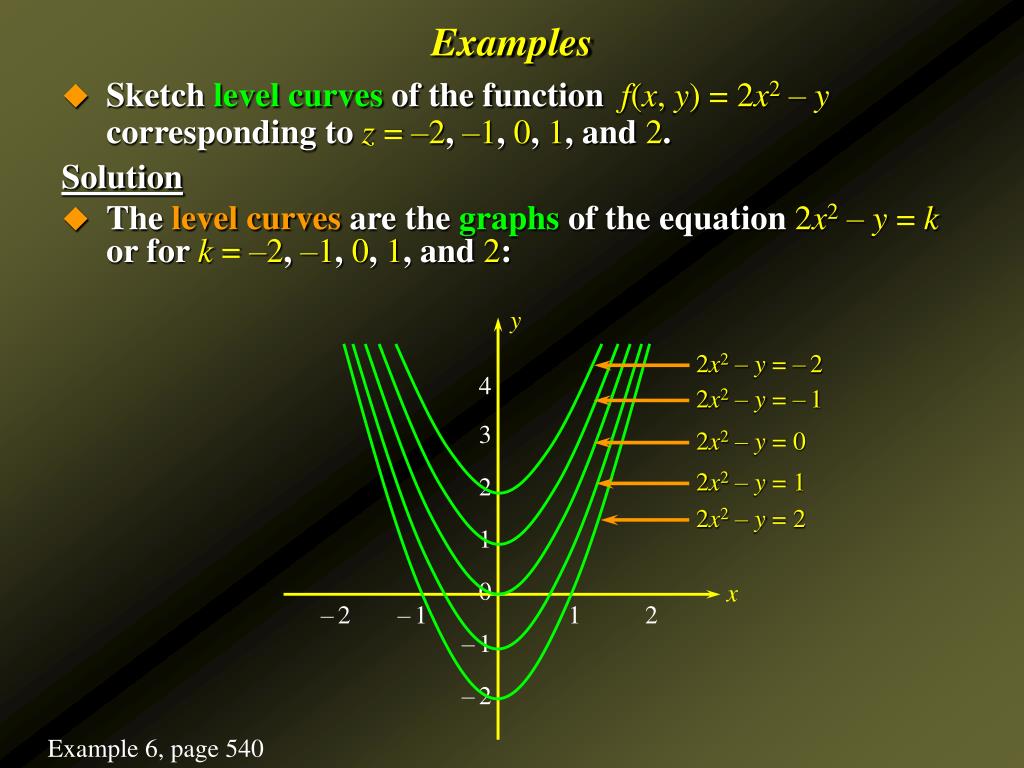
(e) Below is the graph of z = x2 y2 On the graph of the surface, sketch the traces that you found in parts (a) and (c) For problems 1213, nd an equation of the trace of the surface in the indicated plane Describe the graph of the trace 12 Surface 8x 2 y z2 = 9;Question X^2 Y^2 Z^2 = 0 In A 3D Graph This problem has been solved!Level Curves and Surfaces The graph of a function of two variables is a surface in space Pieces of graphs can be plotted with Maple using the command plot3dFor example, to plot the portion of the graph of the function f(x,y)=x 2 y 2 corresponding to x between 2 and 2 and y between 2 and 2, type > with (plots);
(b) Z1 0 Zp x x2 p x x2 p x2 y2 dydx Solution (a) Z2 0 Zp 4 x2 0 p x2 y2 dydx = Zˇ=2 0 Z2 0 r2 drd = 4ˇ 3 (b) The region of integration is inside the (x 1=2)2y2Their defining characteristic is that their intersections with planes perpendicular to any two of the coordinate axes are hyperbolas There are two types of hyperboloids the first type is illustrated by the graph of x 2 y 2 z 2 = 1, which is shown in the figure below As the figure at the right illustrates, this shape is very similar to the one commonly used for nuclear power plant coolingHow to plot 3 dimensional graph for x^2 y^2 = 1?
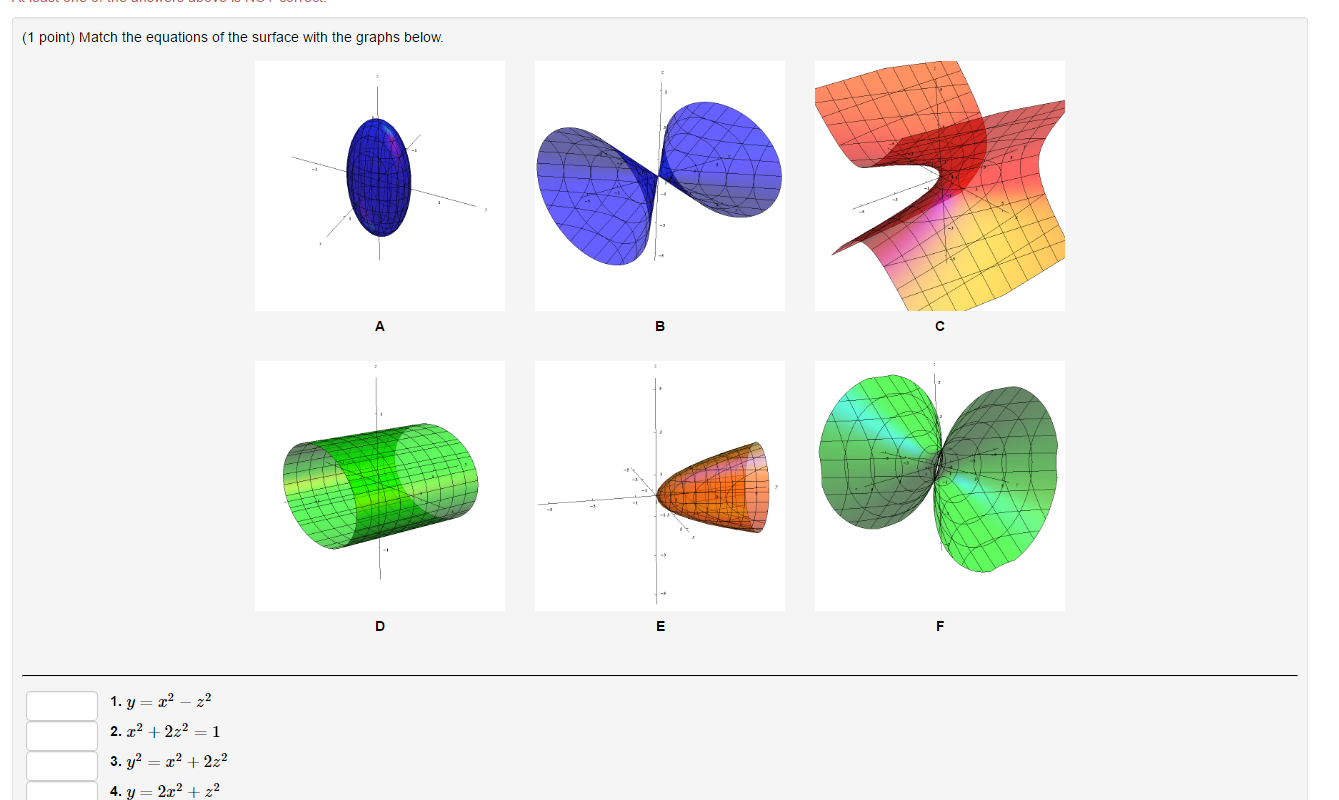


Solved Match The Equations Of The Surface With The Graphs Chegg Com


Some Examples To Show That Objects Be Presented By Mathematical Equations
Calculus Calculus Early Transcendentals Find the points on the hyperboloid x 2 4 y 2 − z 2 = 4 where the tangent plane is parallel to the plane 2 x 2 y z = 5 more_vert Find the points on the hyperboloid x 2 4 y 2 − z 2 = 4 where the tangent plane is parallel to the plane 2 x 2 y z = 5Z= k)x2 y2 k2 = 1 )x2 y2 = 1k2 The trace is a circle whose radius is p 1k2 Therefore the surface is a stack of circles, whose traces of other directions are hyperbola So it is a hyperboloid The intersection with the plane z= kis never empty This implies the hyperboloid is connected (b)If we change the equation in part (a) to x2 y2 z2 = 1, how is the graphPlotting graphics3d Share Improve this question Follow asked Nov 29 '15 at 533 user user
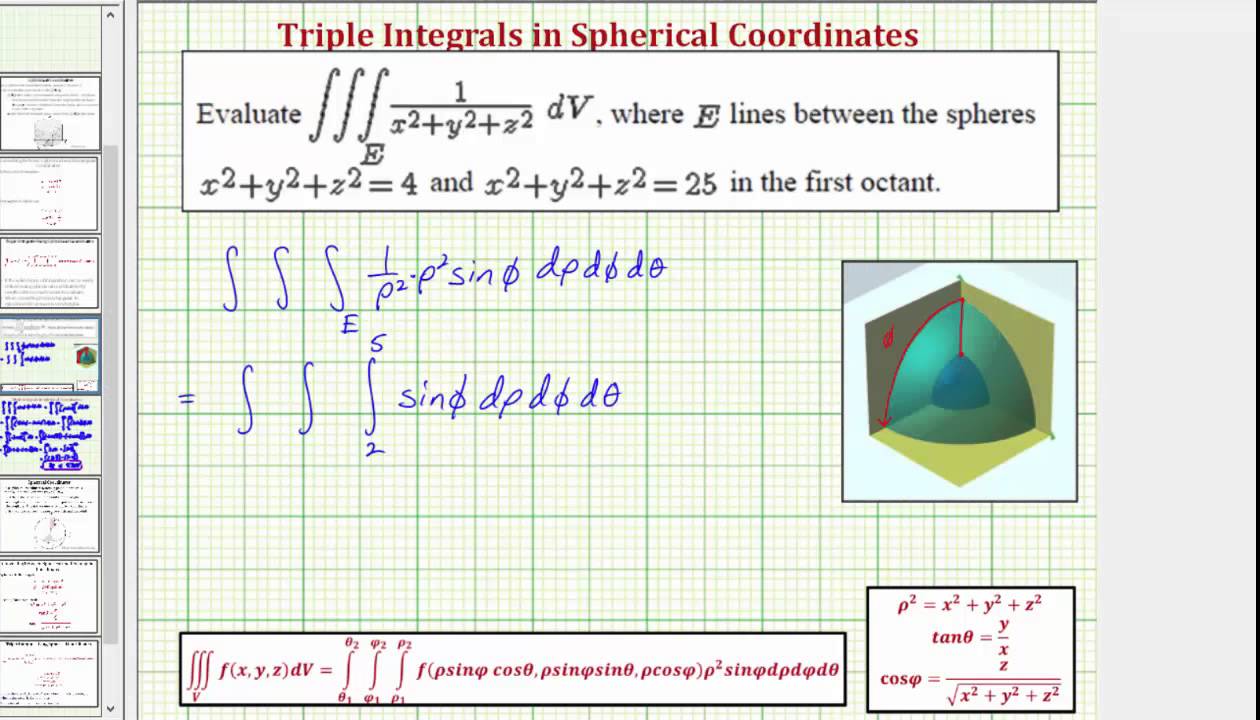


Evaluate A Triple Integral Using Spherical Coordinates Triple Integral Of 1 X 2 Y 2 Z 2 Youtube
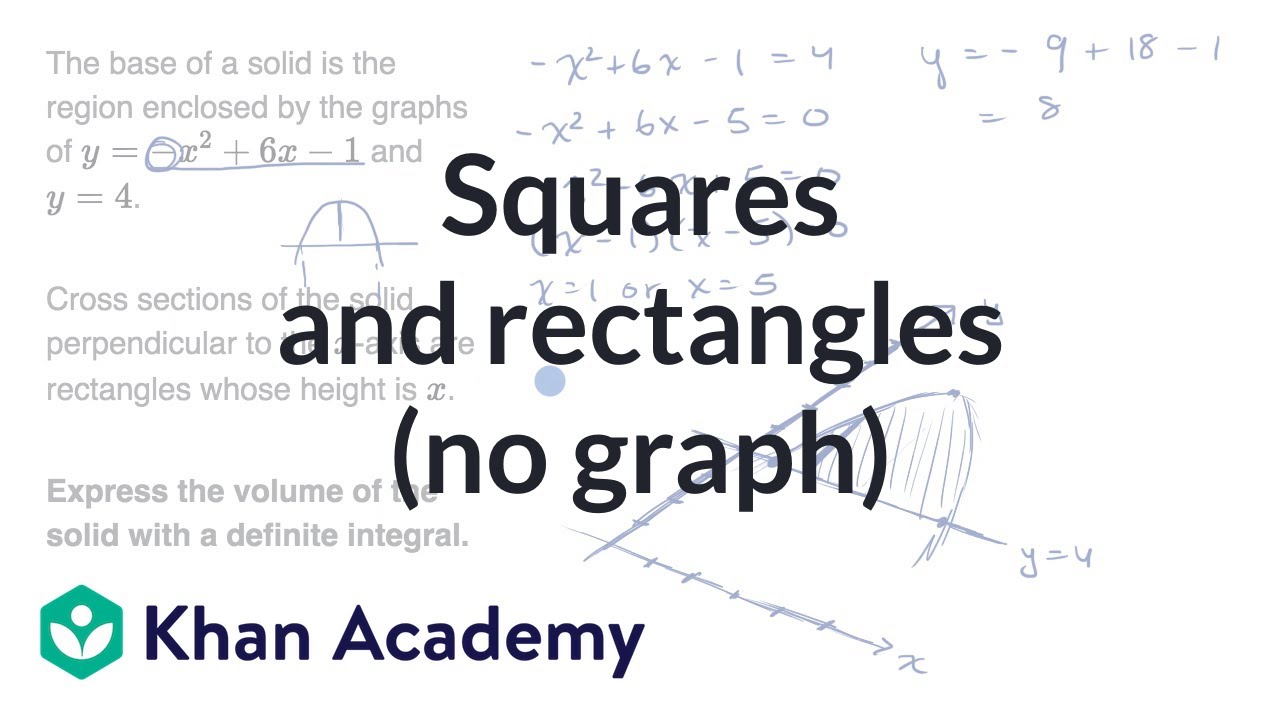


Volume With Cross Sections Squares And Rectangles No Graph Video Khan Academy
Graph x^2y^2=1 This is the form of a circle Use this form to determine the center and radius of the circle Match the values in this circle to those of the standard form The variable represents the radius of the circle, represents the xoffset from the origin, and represents the yoffset from originCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historySurfaces and Contour Plots Part 4 Graphs of Functions of Two Variables The graph of a function z = f(x,y) is also the graph of an equation in three variables and is therefore a surfaceSince each pair (x,y) in the domain determines a unique value of z, the graph of a function must satisfy the "vertical line test" already familiar from singlevariable calculus


The Maximum Value Of Z 5x 4y Subject To Yle2x Xle2y X Yle3 Xge



Choose The Graph Of Y X 3 2 1 Brainly Com
X^2 Y^2 Z^2 = 0 In A 3D Graph;Conic Sections (see also Conic Sections) Point x ^2 y ^2 = 0 Circle x ^2 y ^2 = r ^2 Ellipse x ^2 / a ^2 y ^2 / b ^2 = 1 Ellipse x ^2 / b ^2 y ^2 / a ^2 = 1 Hyperbola x ^2 / a ^2 y ^2 / b ^2 = 1 Parabola 4px = y ^2 Parabola 4py = x ^2 Hyperbola y ^2 / a ^2 x ^2 / b ^2 = 1 For any of the above with a center at (j, k) instead of (0,0), replace each x term with (xj) andX 2 y 2 = z 2 Subtract y^ {2} from both sides Subtract y 2 from both sides x^ {2}=z^ {2}y^ {2} x 2 = z 2 − y 2 Take the square root of both sides of the equation Take the square root of both sides of the equation x=\sqrt {\left (zy\right)\left (yz\right)} x=\sqrt {\left (zy\right)\left (yz\right)}
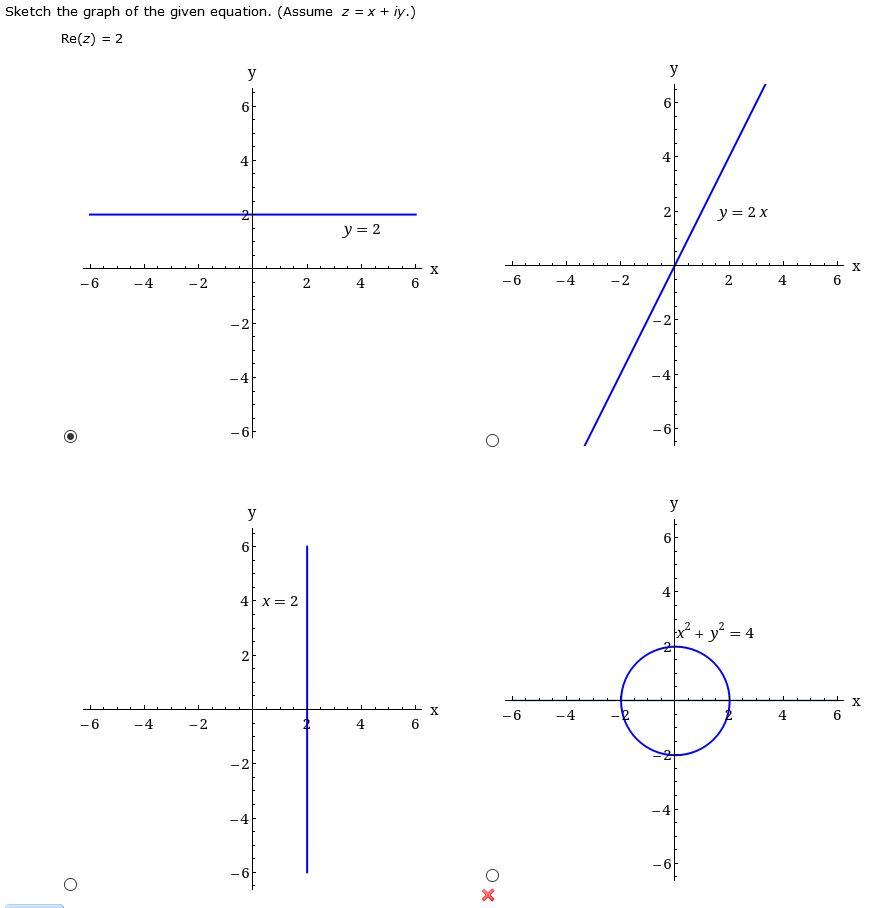


Solved Sketch The Graph Of The Given Equation Assume Z Chegg Com
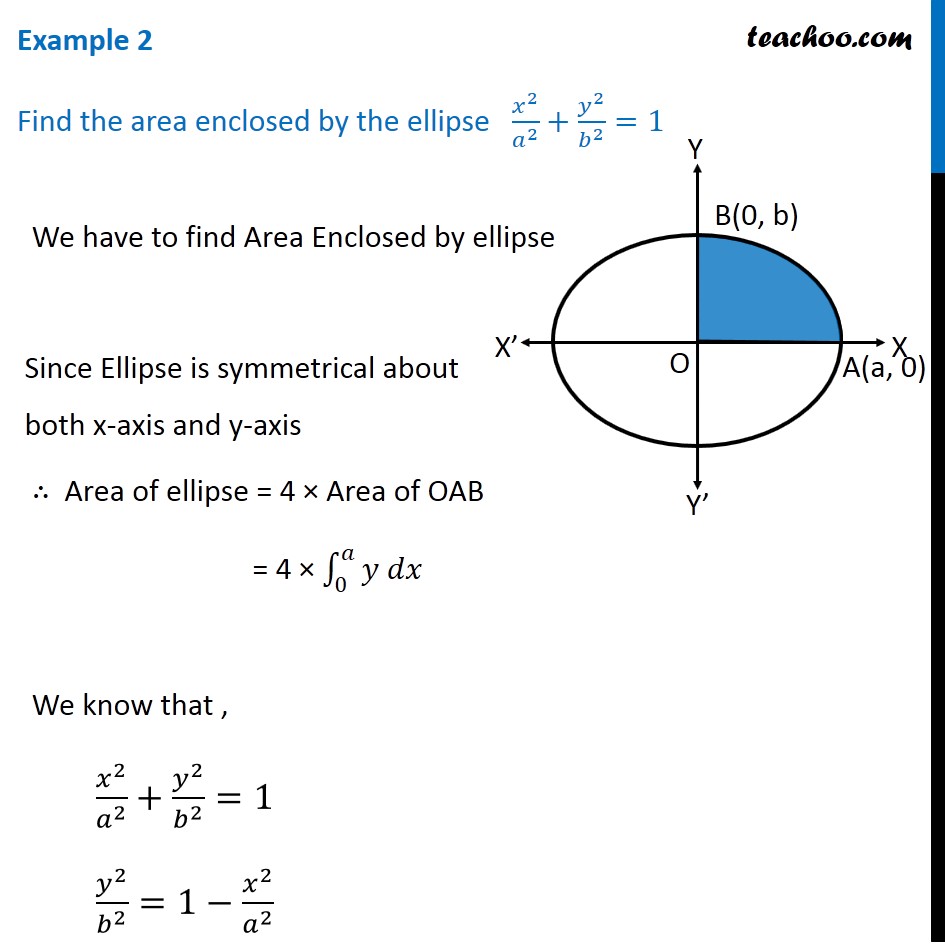


Example 2 Find Area Enclosed By Ellipse X2 Y2 B2 1
Graph x^2y^2=1 Find the standard form of the hyperbola Tap for more steps Flip the sign on each term of the equation so the term on the right side is positive Simplify each term in the equation in order to set the right side equal to The standard form of an ellipse or hyperbola requires the right side of the equation beSketch the new graph TABLE 2 Graphs of quadric surfacesX 2 y 2 z 2 1 2 z 2 x 2 y 2 3 x 2 y 2 z 2 1 4z x 2 y 2 5z 2 x 2 y 2 1 correct 6 from M427L 427L at University of Texas This preview shows page 10 13 out of 13 pagespreview shows page 10


Ppt Functions Of Several Variables Partial Derivatives Powerpoint Presentation Id


How Do I Plot A Function Under Some Constrainets Mapleprimes
Plane z = 1 The trace in the z = 1 plane is the ellipse x2 y2 8 = 1, shown below 6The function is de ned for x 2 y 1 0 and 4 x2 y2 0 The domain is D = f(x;y) 2R2j1 x2 y2 < 4g This is an annular region in the xyplane (b) f(x;y) = p x p y p x2 y2 16 The function is de ned for x 0, y 0, and x2 y2 16 0 The domain is D = f(x;y) 2R2jx 0;y 0;x2 y2 16g This is the portion of the region outside the circle centeredZ 2 1 something dz To write the inner double integral, we want to describe each slice (and, within a slice, we can think of zas being a constant) Each slice is just the disk enclosed by the circle x2 y2 = z2, which is a circle of radius z (2)This is true because z= p 3(x 2 y ) can be written in cylindrical coordinates as r p 3


Andrew Parker S Fabulous Matlab Graphs


Taak Vraag 1 En 2 Geogebra
2 We can describe a point, P, in three different ways Cartesian Cylindrical Spherical Cylindrical Coordinates x = r cosθ r = √x2 y2 y = r sinθ tan θ = y/x z = z z = z Spherical Coordinates1 This figure is the (double) cone of equation x 2 = y 2 − z 2 The gray plane is the plane ( x, y) You can see that it is a cone noting that for any y = a the projection of the surface on the plane ( x, z) is a circumference of radius a with equation z 2 x 2 = a 2Conic Sections Parabola and Focus example Conic Sections Ellipse with Foci



Surface Area Of Z X 2 Y2 1 2 Youtube



Koval S 3d Grapher
The graph z = x2 y2 of the function f(x,y) = x2 y2 which is a paraboloid Note however that most surfaces of the form g(x,y,z) = c can not be written as graphs The sphere is an example, where we need two graphs to cover it Planes ax by cz = d is a plane With ~n = ha,b,ci and ~x = hx,y,zi, we can rewrite theSee the answer Show transcribed image text Expert Answer 100% (1 rating) Previous question Next question Transcribed Image Text from this Question x^2 y^2 z^2 = 0 in a 3D graphSketch the new graph
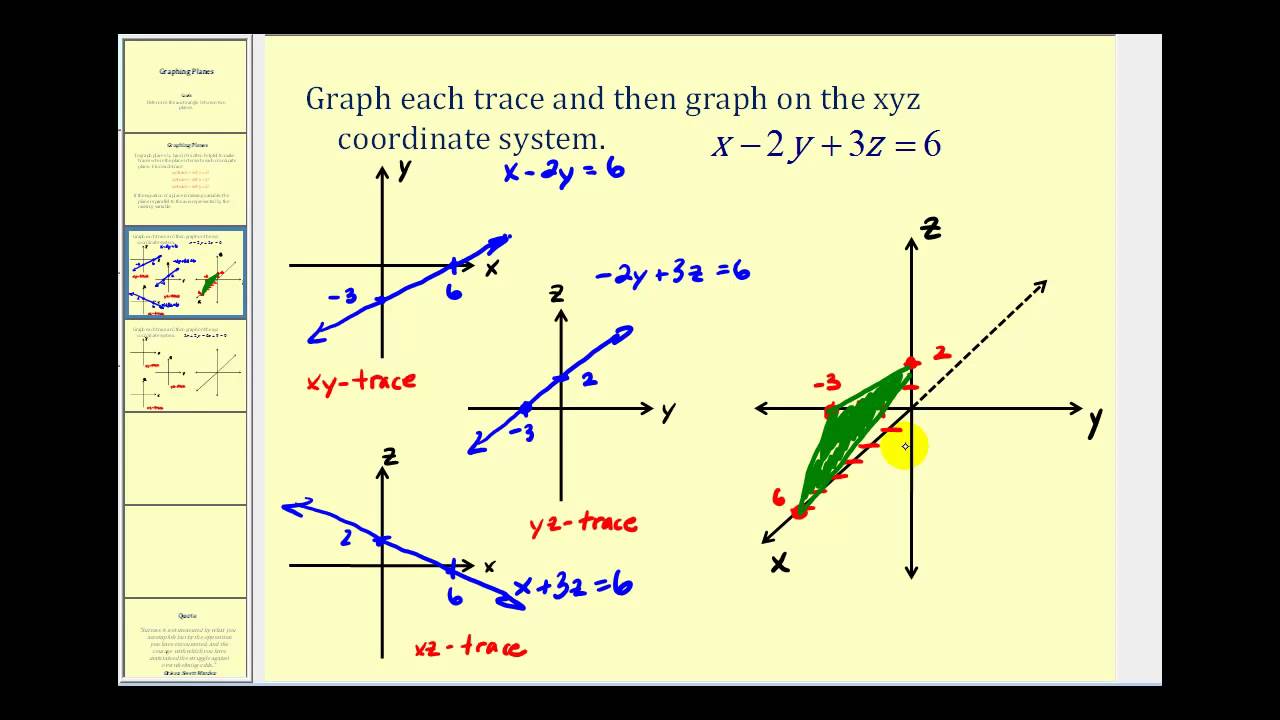


Graphing A Plane On The Xyz Coordinate System Using Traces Youtube



Quadratic Function Wikipedia
Jan 24, 16 · It is the equation of a circle Probably you can recognize it as the equation of a circle with radius r=1 and center at the origin, (0,0) The general equation of the circle of radius r and center at (h,k) is (xh)^2(yk)^2=r^2X2 y2)dA = Z2ˇ 0 Z2 1 (sinr)rdrd = 2ˇ(cos(1) 2cos(2)sin(2) sin(1)) 2 Calculate the following integrals by changing to polar coordinates (a) Z2 0 Zp 4 x2 0 p x2 y2 dydx;To use the application, you need Flash Player 6 or 7 Click below to download the free player from the Macromedia site Download Flash Player 7


Answer In Calculus For Ero



Solved Question 3 10 Pts 1 Match Each Graph With Its Chegg Com
In the twodimensional coordinate plane, the equation x 2 y 2 = 9 x 2 y 2 = 9 describes a circle centered at the origin with radius 3 3 In threedimensional space, this same equation represents a surface Imagine copies of a circle stacked on top of each other centered on the zaxis (Figure 275), forming a hollow tubeNov 29, 15 · (a) Find and identify the traces of the quadric surface – x 2 – y 2 z 2 = 1 and explain why the graph looks like the graph of the hyperboloid of two sheets in Table 2 (b) If the equation in part (a) is changed to x 2 – y 2 – z 2 = 1, what happens to the graph?2 21 Functions and graphs A function f Rn → Rm send each point x ∈ Rn to a specific point f(x) ∈ Rm If m = 1 it is called a scalar valued function and if m > 1 a vector valued function Ex The temperature at each point in space is a scalar function (or field) fR3 →R Ex The velocity of a fluid is a vector that depends on the point in space as well as


Multiple Integrals H 2 Y Are Continuous Functions On C D And Let F X Y Be A Function Defined On R Then Pdf Free Download



Which Area Is Referred To By Dxdy In D X Y Z 0 Leq Z Leq X 2 Y 2 Leq X 2 Y 2 Leq 1 Y Geq X Mathematics Stack Exchange
Find the equation of the sphere which touches the sphere x^2y^2z^22x6y1=0 at (1,2,2) and pass through (1,1,0)Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyBox next to the graph (2pointseach) (A) ¡y2 sin(x) (B) 1 1¯x2 ¯y2 7 Consider the function g R2!R whose graph is shown at right Let A and B be the points in R2 corresponding to two "peaks" of the graph, and C be the point in R2 corresponding to the dot on the graph For each part, circle the answer that is most consistent with the


12 6 Quadric Surfaces Mathematics Libretexts



Draw The Graph Of The Following Pair Of Linear Equations X 3y 6 2x 312 Hence Find The Area Of Region Bounded By Lines X 0 Y 0 And 2x 3y 12
Feb 12, 17 · how can i draw graph of z^2=x^2y^2 on matlab Follow 245 views (last 30 days) Show older comments Rabia Kanwal on 12 Feb 17 Vote 0 ⋮ Vote 0 Commented Walter Roberson on 10 Mar Accepted Answer Star Strider 0 Comments Show Hide 1 older comments Sign in to comment Sign in to answer this questionSee the answer Show transcribed image text Expert Answer 100% (1 rating) Previous question Next question Transcribed Image Text from this Question10 {eq}z^2 = x^2y^2 {/eq} Graph Description of data or restructure the data and information in the form of a diagram, chart, picture, and picturesque representation is termed as a graph



32 Linearization Pdf Plane Geometry Gradient


Quadratic Functions And Their Graphs
Sep 04, 07 · For any value of z, it clearly has a circular crosssection (parallel to the (x, y) plane, of course), since x and y only appear in the combination x^2 y^2 Once you realize it has circular symmetry around the zaxis, it is preferable to switch from rectangular Cartesian coordinates (x, y, z) to cylindrical coordinates (r, φ, z), where x = r cos φ and y = r sin φXz plane traces create ellipses This tells us the surface is an ellipsoid because there are 2 ellipsoid graphs to choose from, we look at the major axis in the function and pick the graph with the corresponding major axis x axis radius = (sqrt (1/9))^2, y axis radius = (sqrt (1/4))^2 z axis radius = 1 We see the major axis is the Z axis, and the corresponding graph is IVAug 30, 15 · (delw)/(delx) = x/sqrt(x^2 y^2 z^2) (delw)/(dely) = y/sqrt(x^2 y^2 z^2) (delw)/(delz) = z/sqrt(x^2 y^2 z^2) Since you're dealing with a multivariable function, you must treat x, y, and z as independent variables and calculate the partial derivative of w, your dependent variable, with respect to x, y, and z When you differentiate with respect to x, you treat y and z as



Se11f01 01 Gif
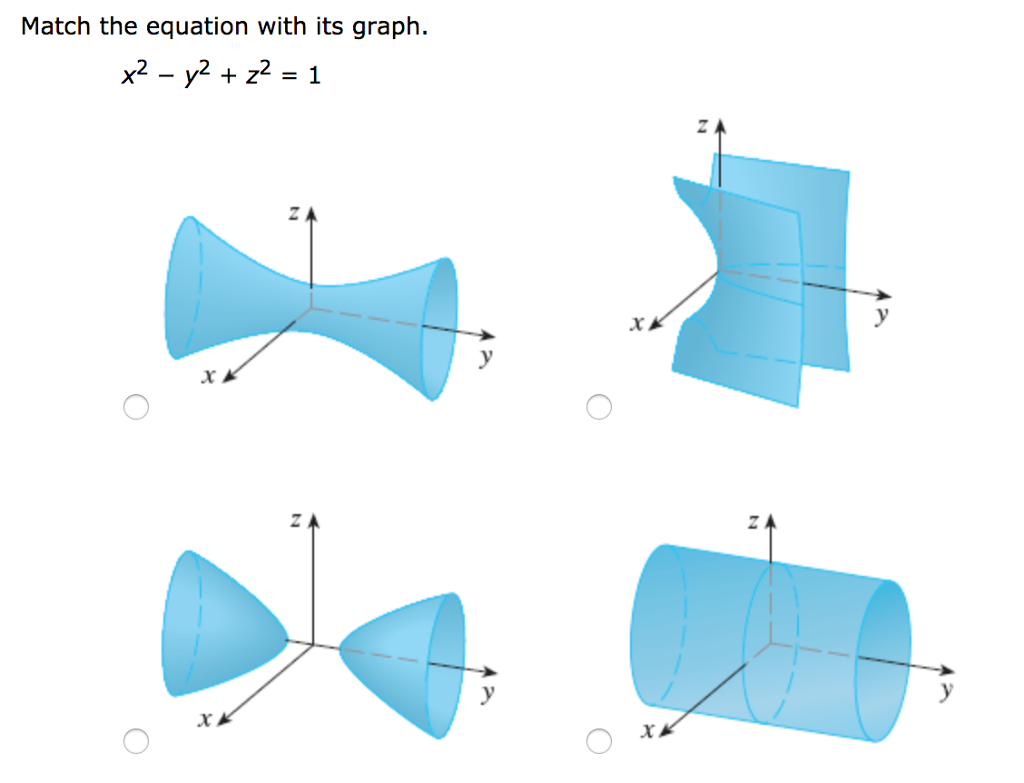


Solved Match The Equation With Its Graph X2 Y2 Z2 1 Chegg Com
The simple way to graph y = x1 is to generate at least two points, put those on your graph paper and draw a straight line through them Here's how you geneate the required points Use your equation, y = x1 and choose an integer for x, say x=2, and substitute this into your equation to find the corresponding value of y y = 2 1 y = 1Question Match The Equation With Its Graph X^2 Y^2 Z^2 = 1 Match The Equation With Its Graph x^2 Y^2 Z^2 = 1 This problem has been solved!If one chooses a cartesian coordinate system, such that the origin is the center of the ellipsoid, and the coordinate axes are axes of the ellipsoid, the implicit equation of the ellipsoid has the standard form x 2 a 2 y 2



Plotting Sympy 1 8 Documentation



How Do You Find An Equation For The Line Tangent To The Circle X 2 Y 2 25 At The Point 3 4 Socratic
I am already using it and I only can plot in 2 dimensional graph Can someone help me with this problem?Feb 15, 16 · 3Dplot of "x^2y^2z^2=1" Learn more about isosurface;Nov 16, 13 · z^2 = x^2y^2 if you slice parallel to the xy plane you will will get a series of circles centered on the z axis if you slice along yz plane ie x = 0 z^2 = y^2 z = y and z = y similarly if y = 0 z = x and z = x you could convert to polar coordinates x = r cos t y = r sin t z^2 = r^2 r = z or r=z (r is > 0 in the conversion to polar coordinates)


0 3 Visualizing Functions Of Several Variables



How To Plot Coordinates c Bitesize
Here is a parameterization for that circle by the angle t x = y = cos ( t) 2, z = sin ( t) You can verify that x ( t) 2 y ( t) 2 z ( t) 2 = 1 for all t, so t parameterizes a circle in 3D To get the projection of this circle onto the x z plane, we "zero out" the ycoordinate, and this gives us
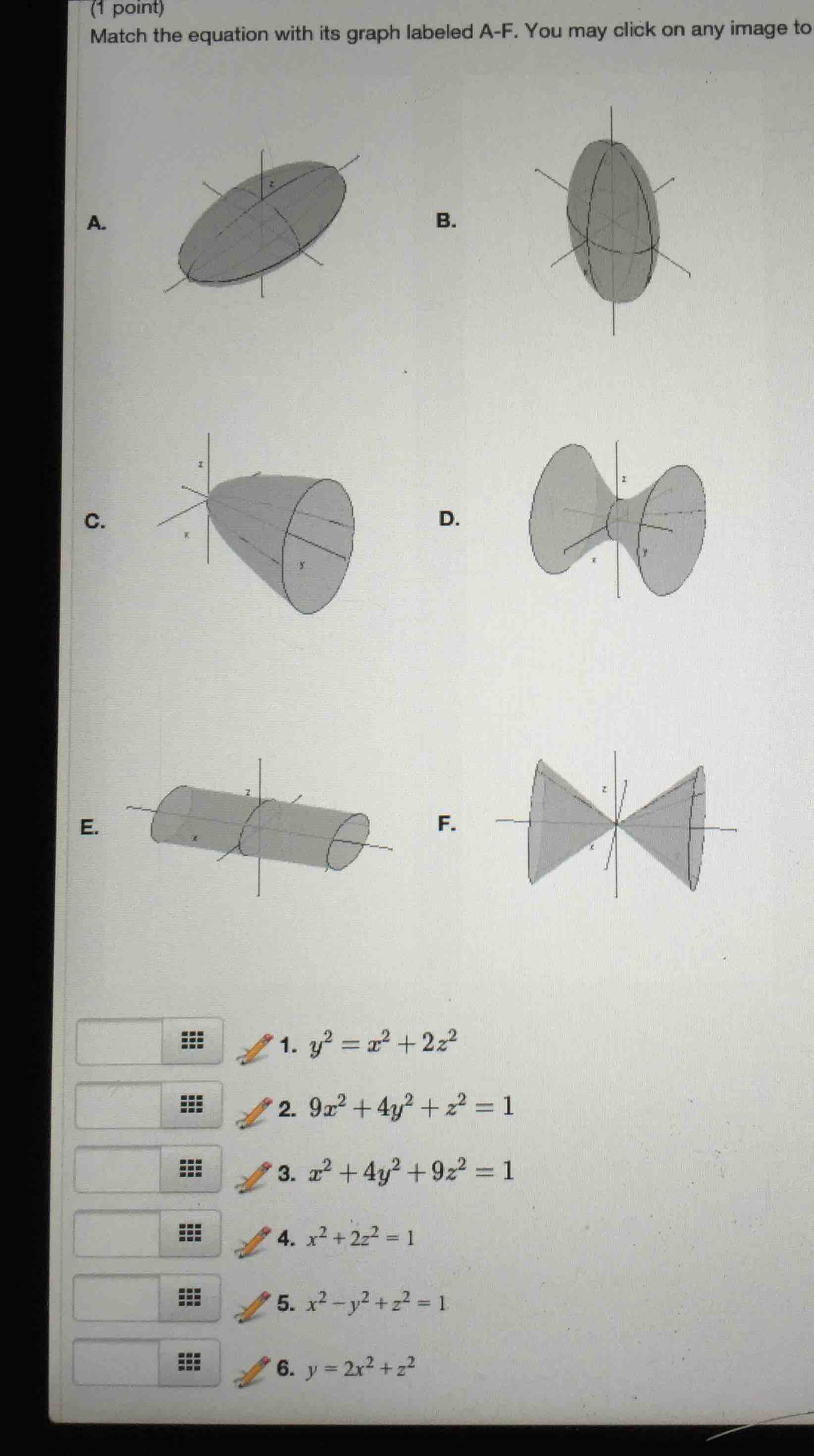


Solved Match The Equation With Its Graph Labeled A F You Chegg Com


I Just Noticed That They Added 3d Graphs How Long Has This Been A Thing Google
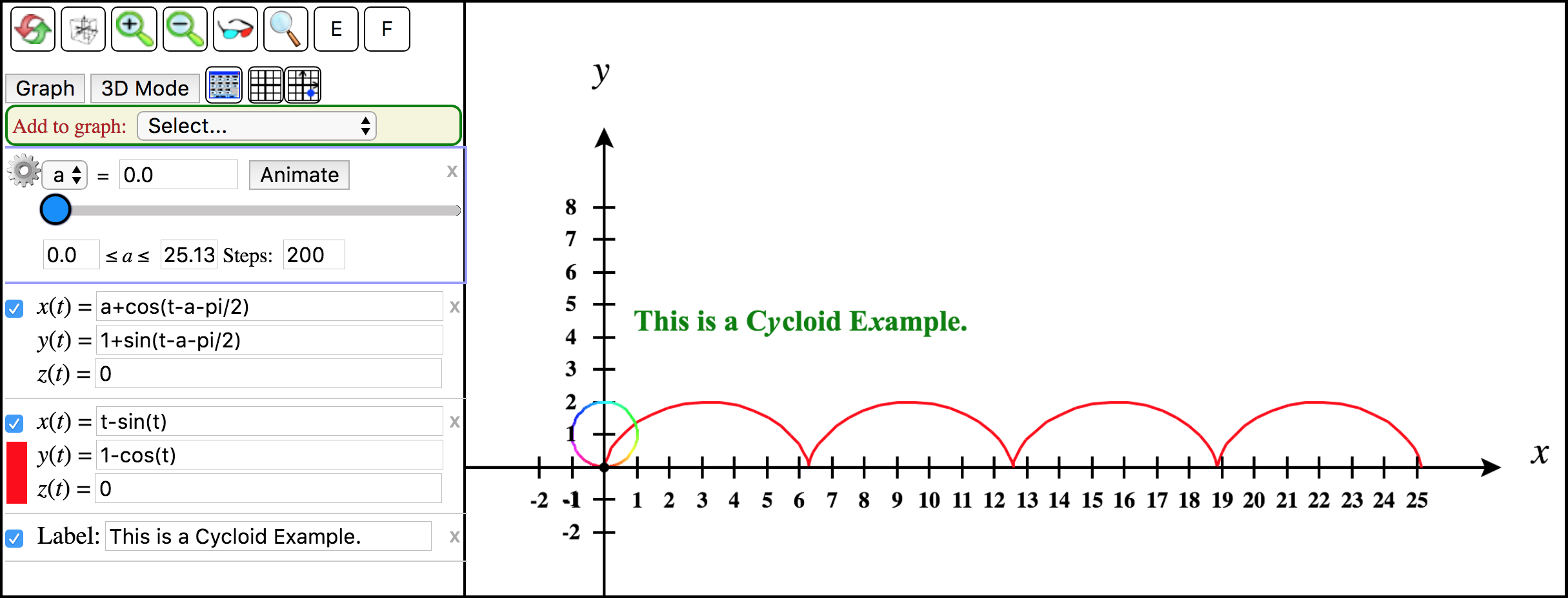


Parameter Sliders



Which Of The Following Graphs Represents The Equation Y 2 3 X 1 Brainly Com



Plotting Surfaces Colgate Pages 1 4 Flip Pdf Download Fliphtml5



Graph X 2 Y 2 4 Youtube
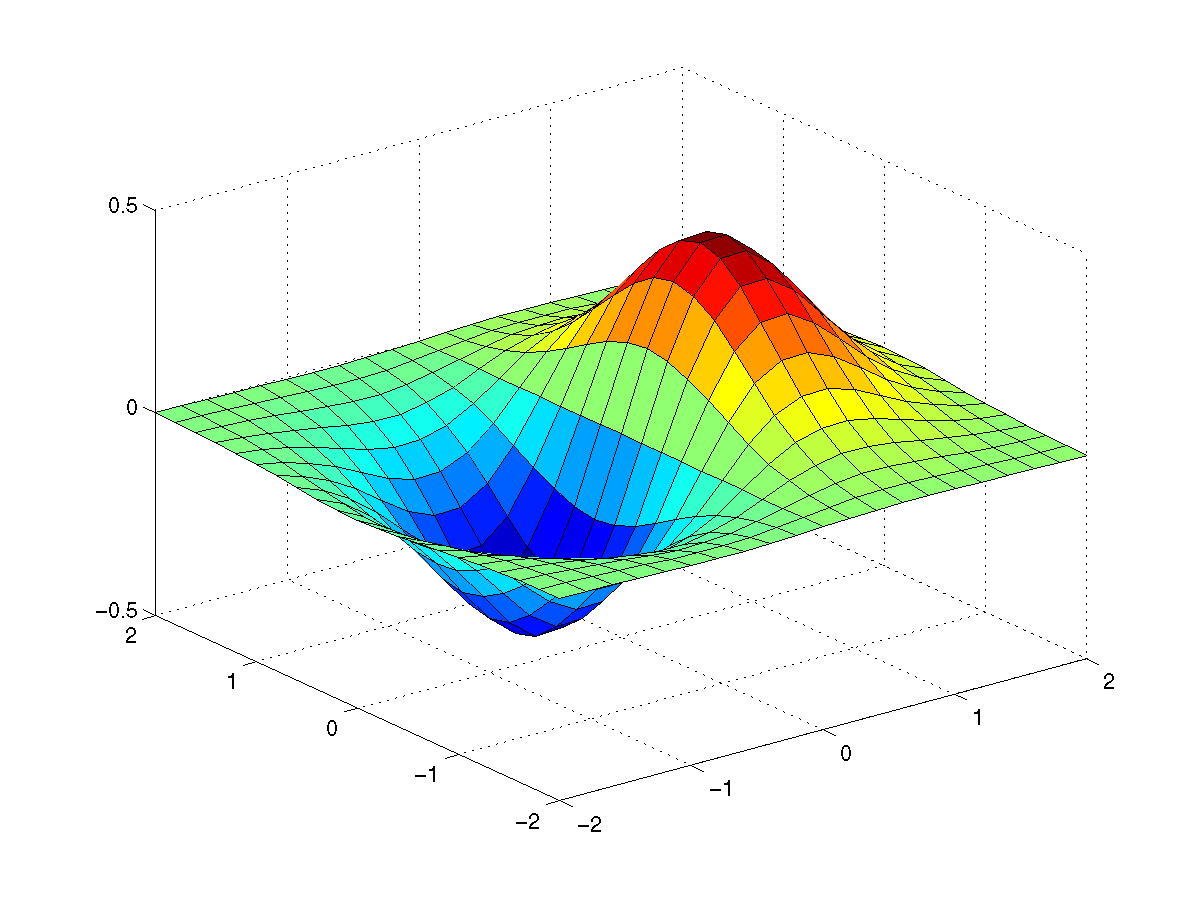


Matlab
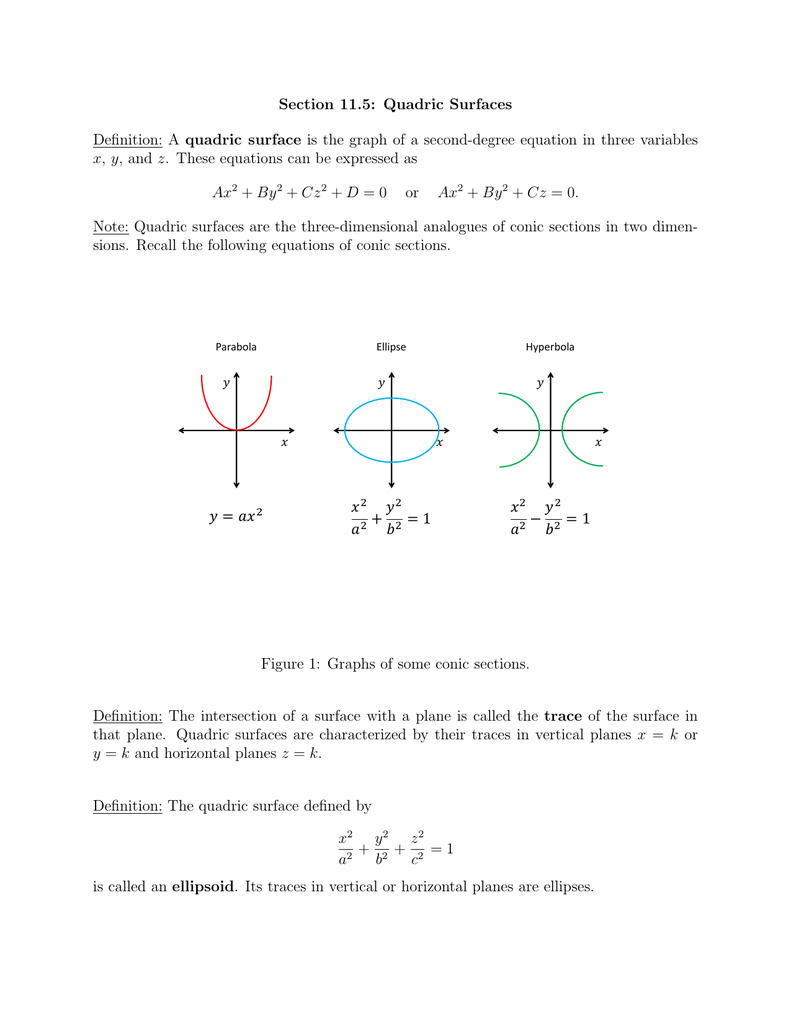


Section 11 5 Quadric Surfaces



Plotting Sympy 1 8 Documentation
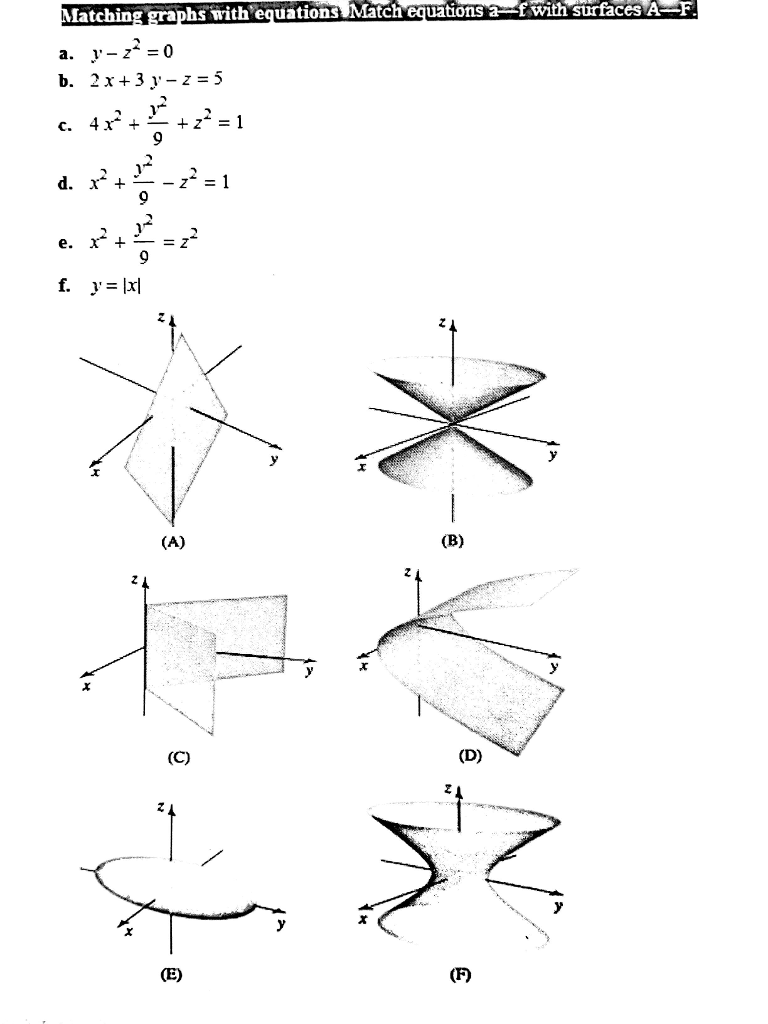


Solved Matching Graphs With Equations Match Equations A F Chegg Com
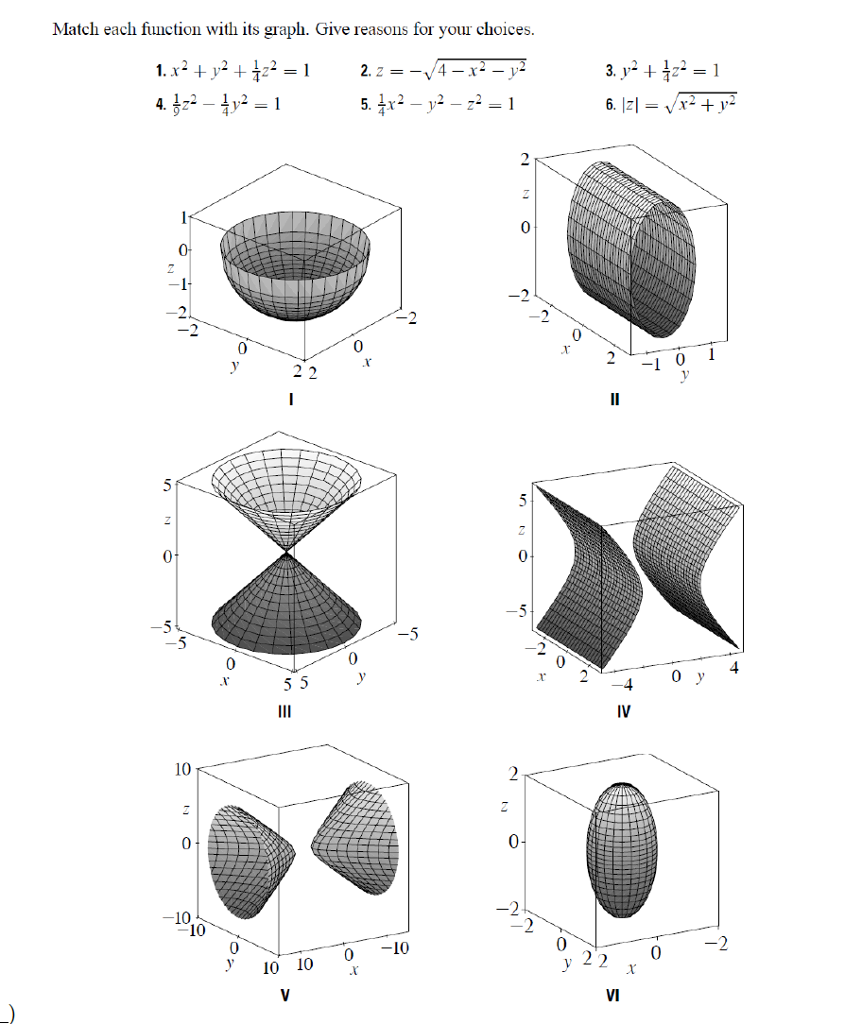


Solved Match Each Function With Its Graph Give Reasons F Chegg Com
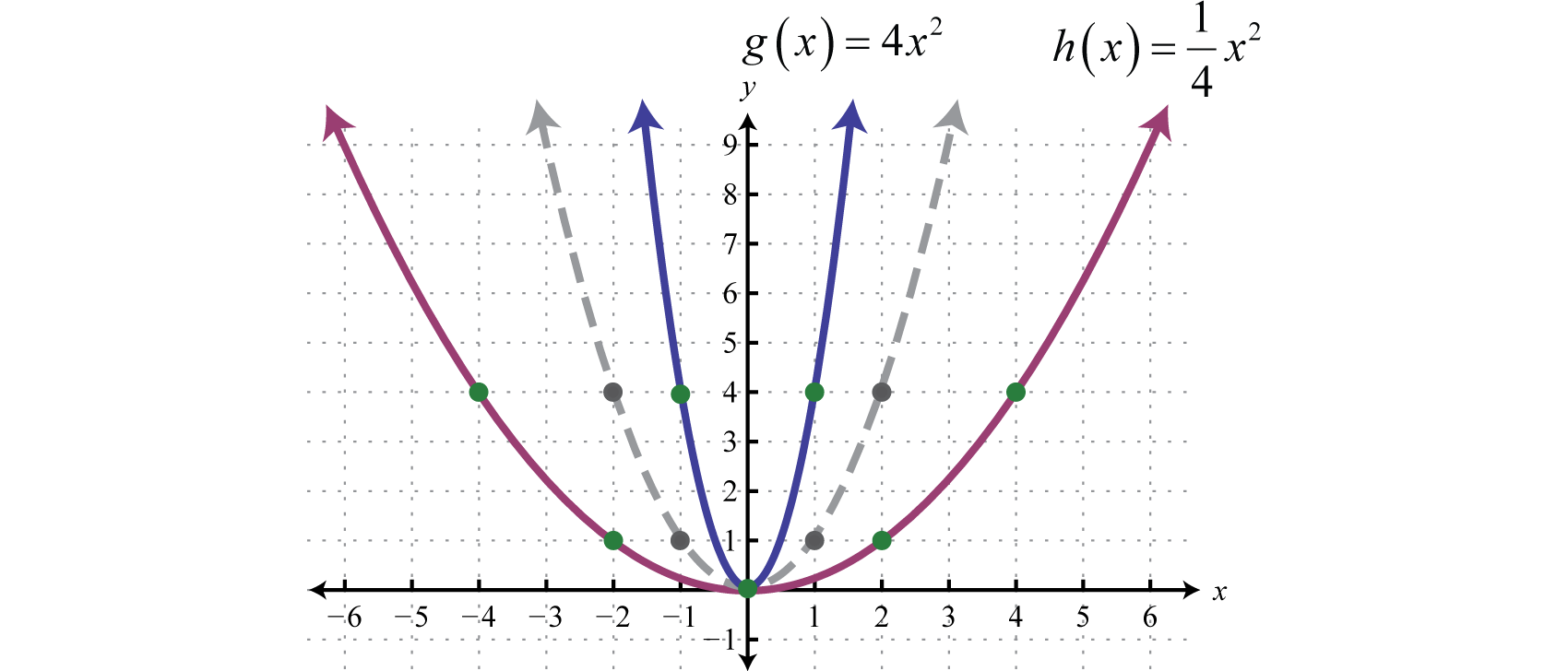


Using Transformations To Graph Functions



Which Graph Represents The Solution Of The System X 2 Y 2 4 X Y 1 Brainly Com
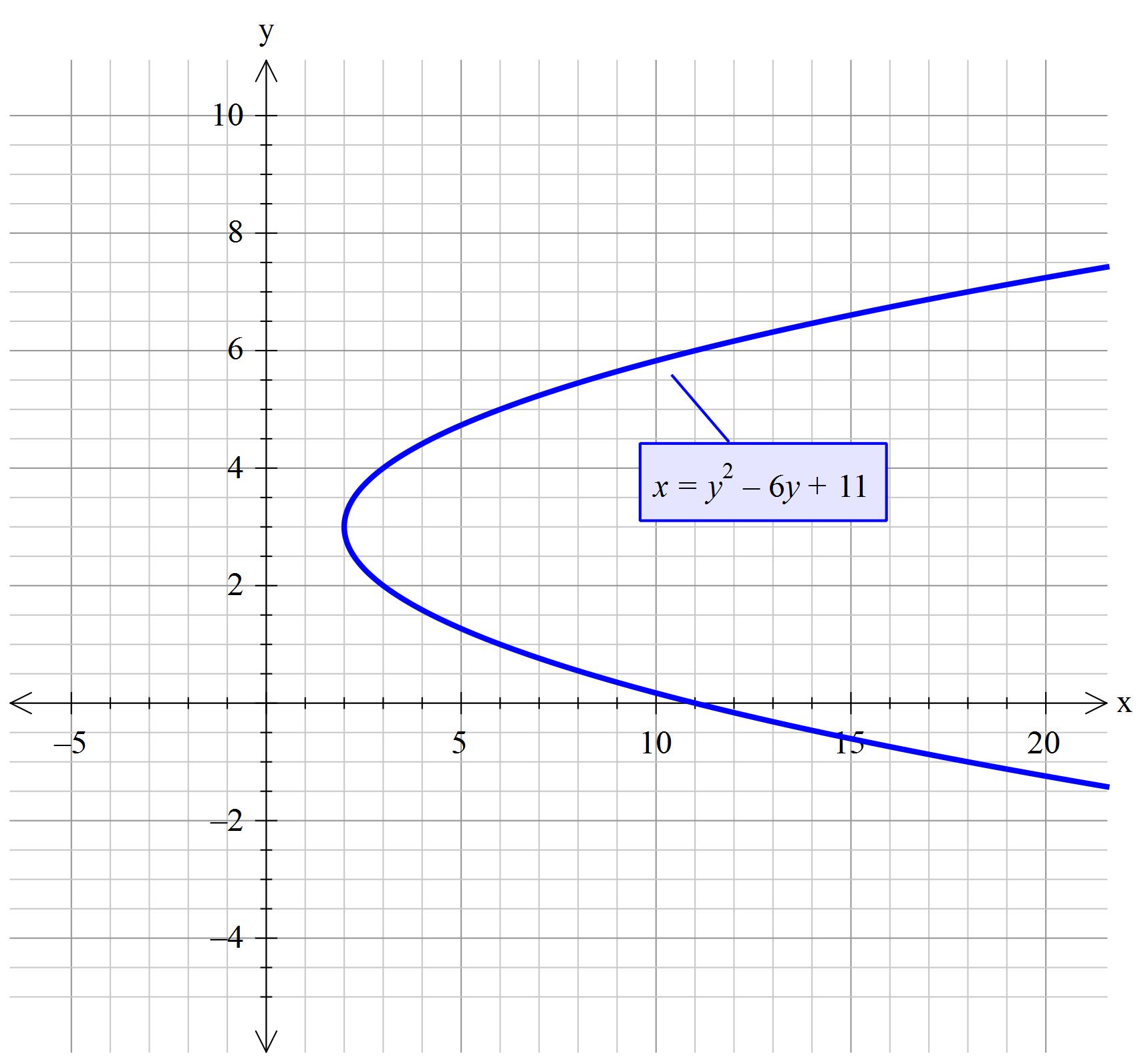


How Do You Graph Y Sqrt X 2 3 Socratic
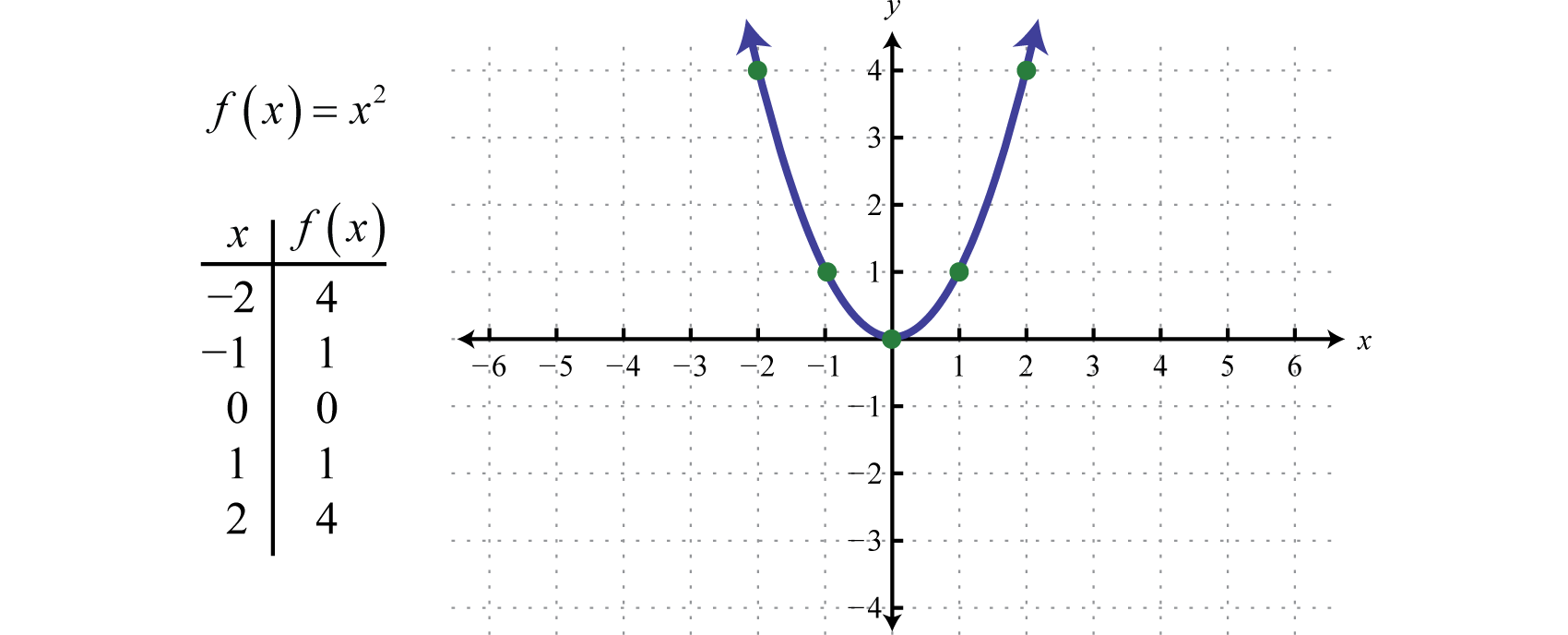


Quadratic Functions And Their Graphs
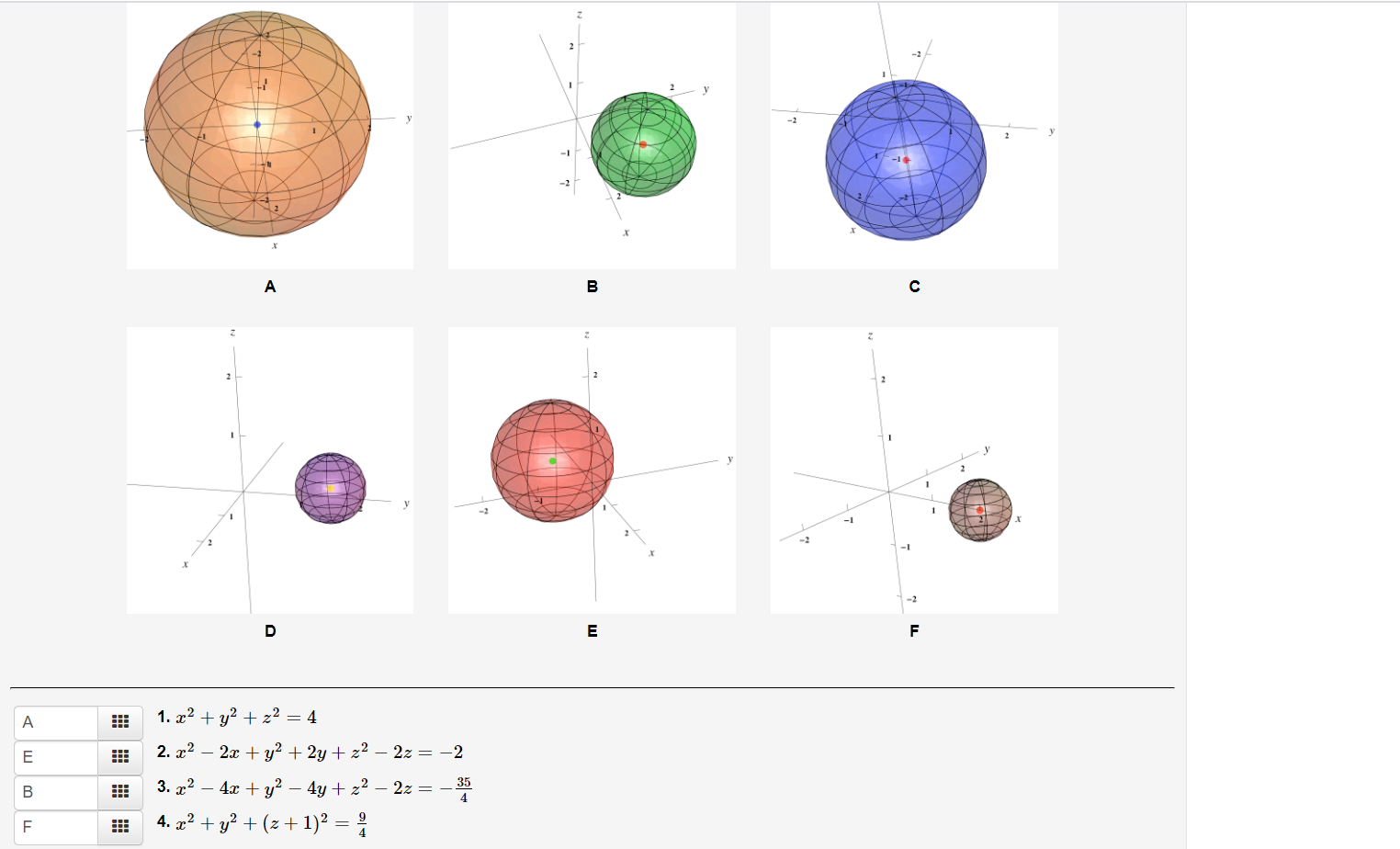


Solved Match The Equations Of The Spheres With One Of The Chegg Com


Practice 3 7



Calc 501 1000 By James Bardo Issuu
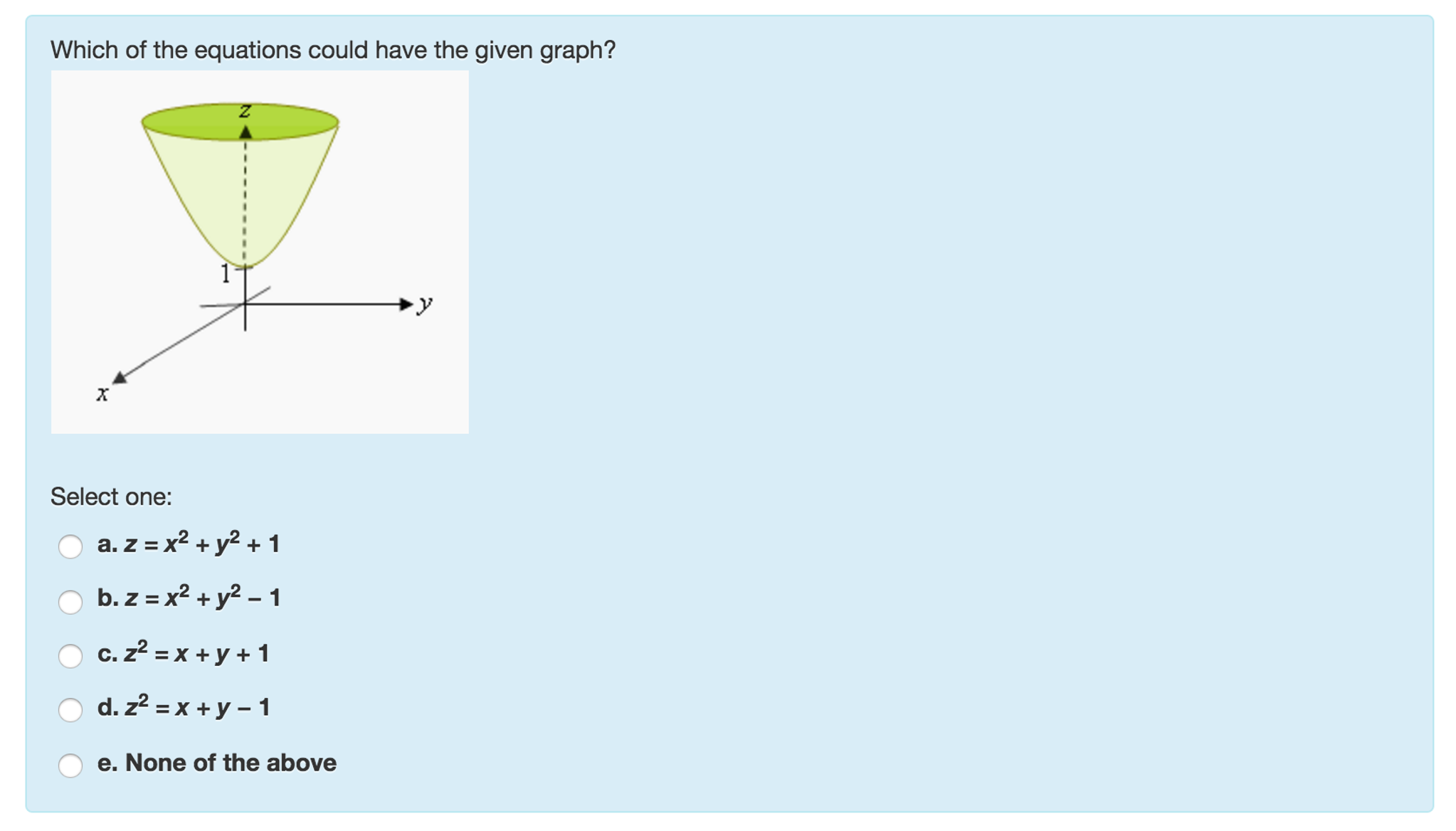


Solved Which Of The Equations Could Have The Given Graph Chegg Com
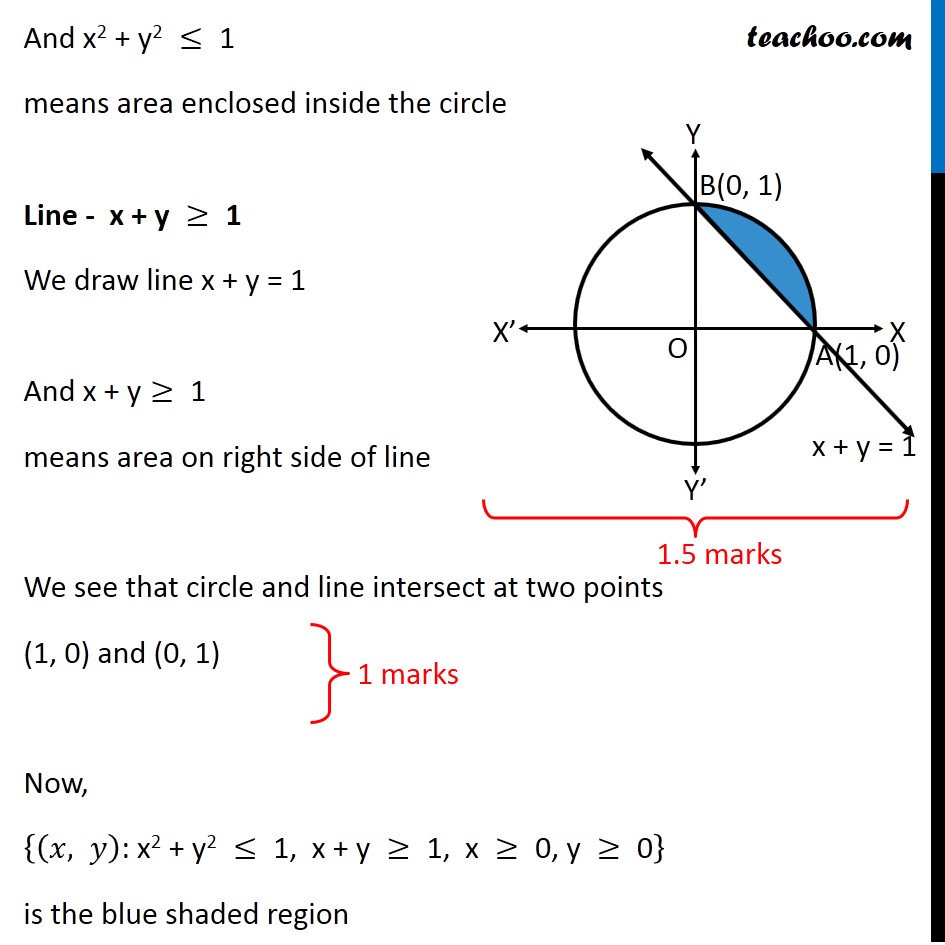


Using Integration Find Area Of Region X Y X 2 Y 2 1 X Y
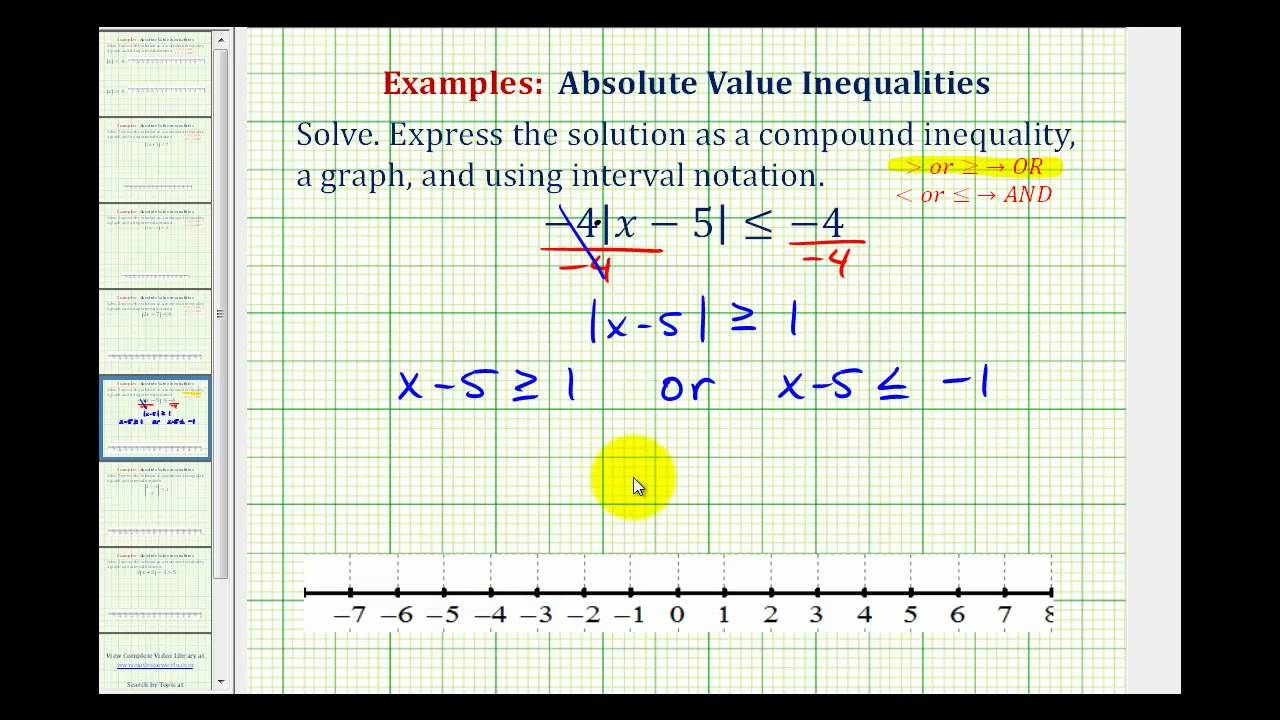


Solve Compound Inequalities Beginning Algebra
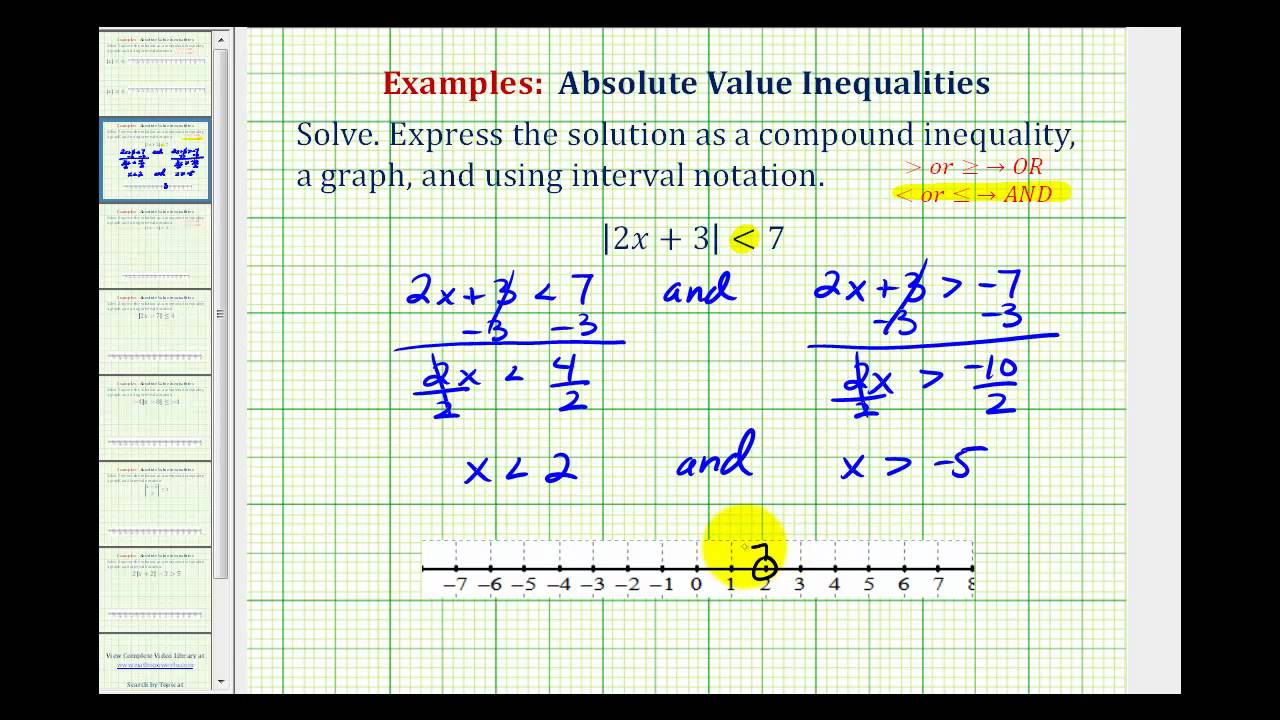


Solve Compound Inequalities Beginning Algebra



How Do You Find The Volume Of The Solid Generated By Revolving The Region Bounded By The Graphs Y 9 X 2 Y 0 X 2 X 3 About The Y Axis Socratic
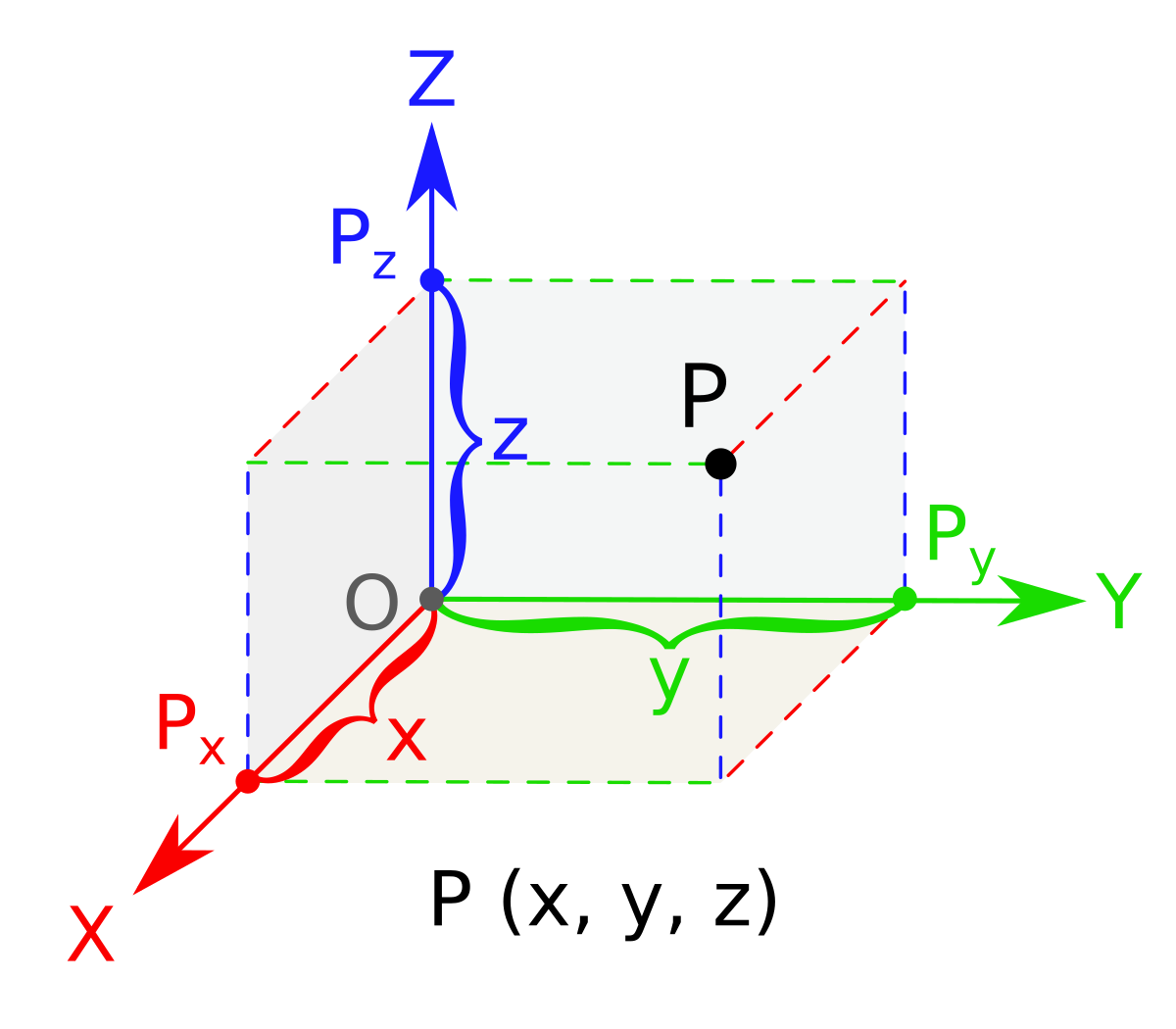


Three Dimensional Space Wikipedia



Graph The Line That Represents This Equation Y 2 1 2 X 2 Brainly Com


12 6 Quadric Surfaces Mathematics Libretexts
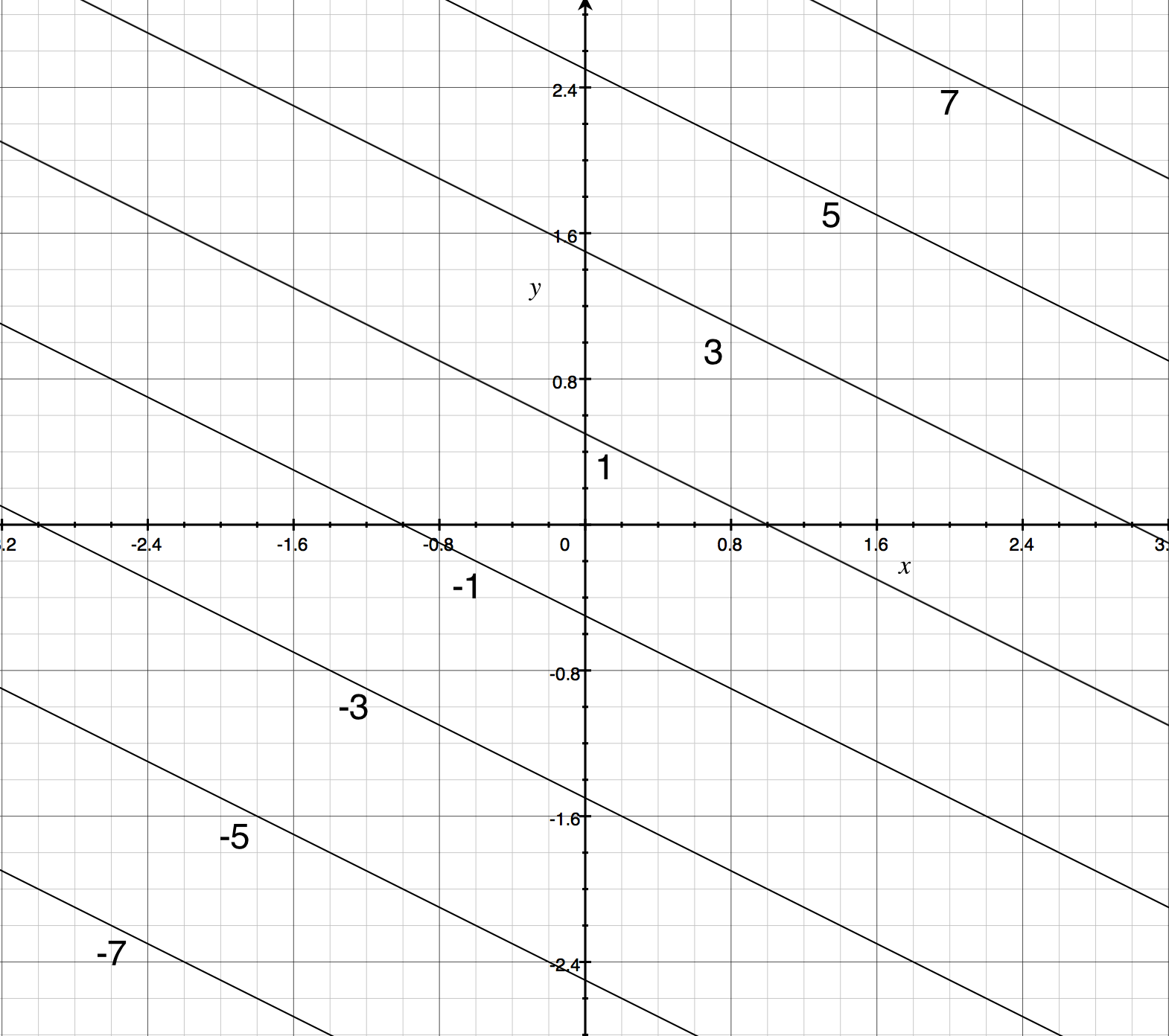


Contour Maps Article Khan Academy
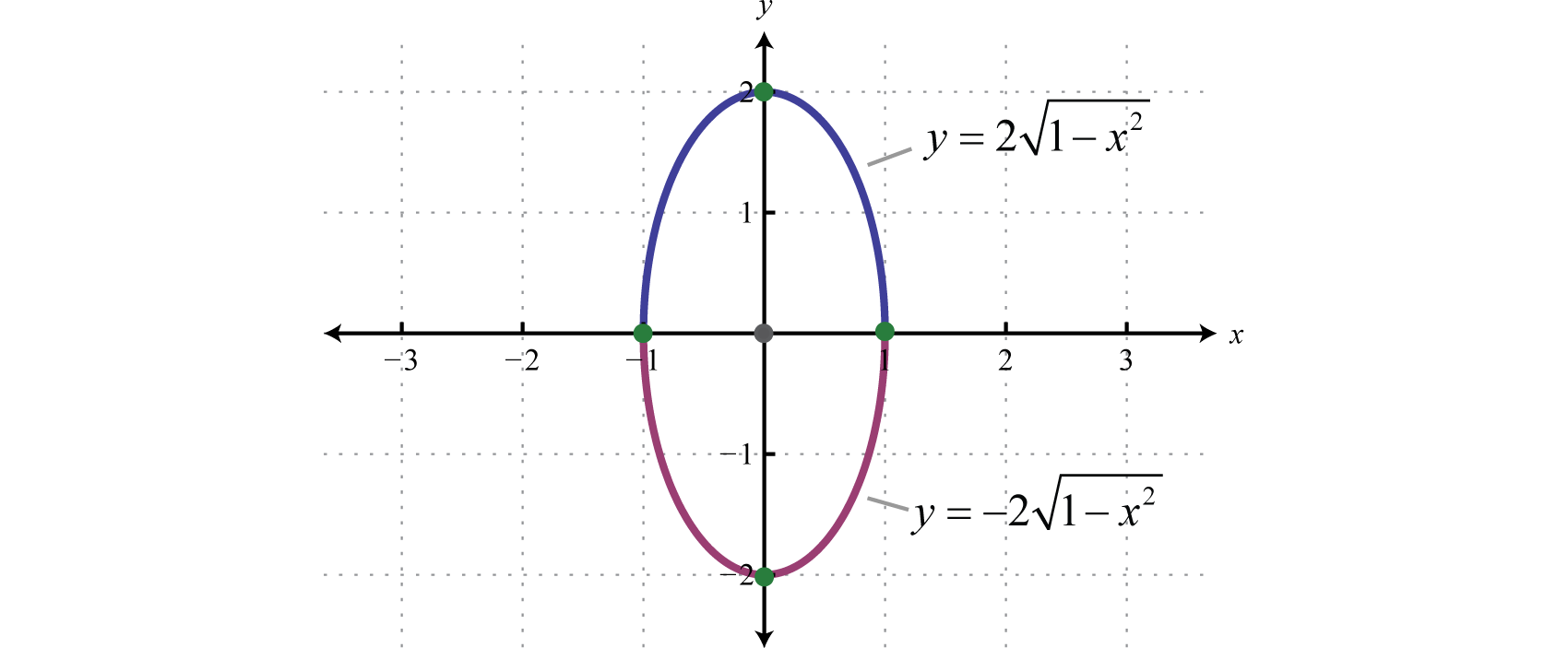


Ellipses
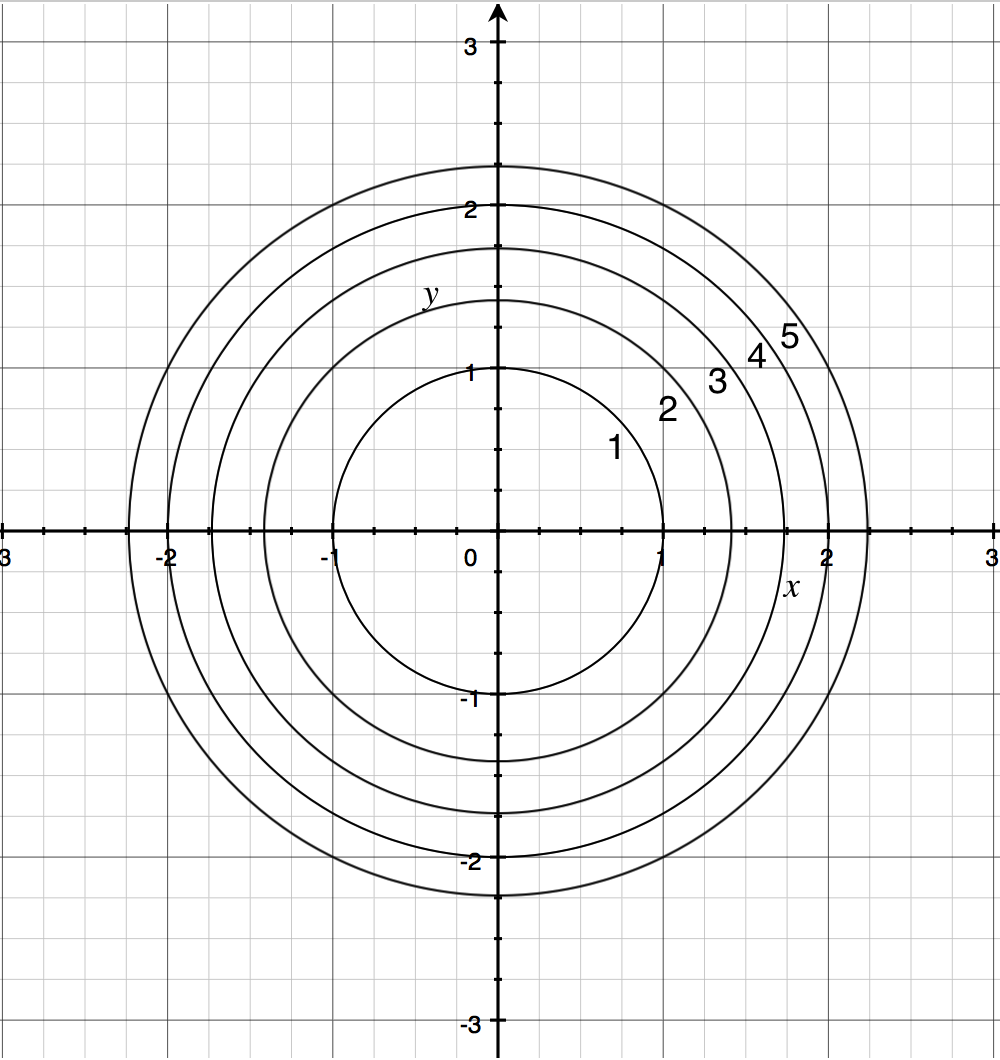


Contour Maps Article Khan Academy
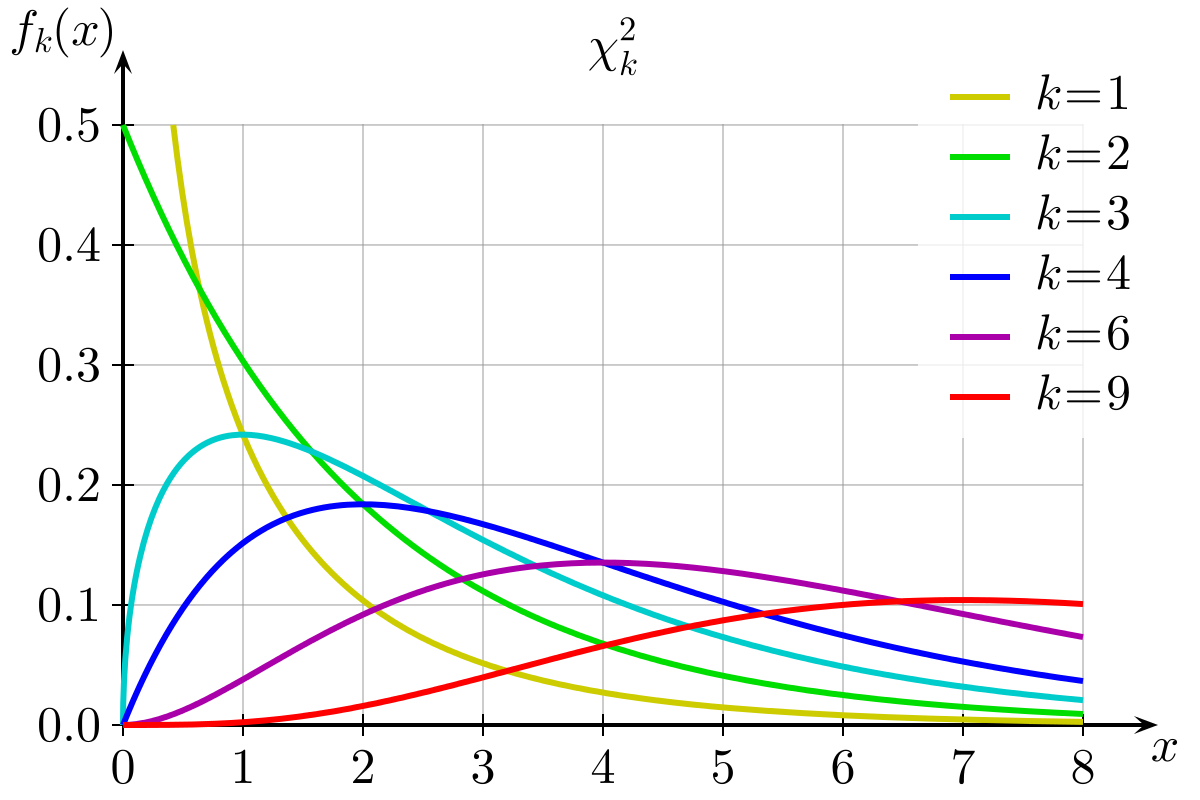


Chi Square Distribution Wikipedia



Triple Integral Boundaries And Solution Mathematica Stack Exchange



If The Plane Containing The Line X 3 2 Y 2 1 Z 1 3



What 3d Graph Does X 2 2z 2 1 Give Mathematics Stack Exchange
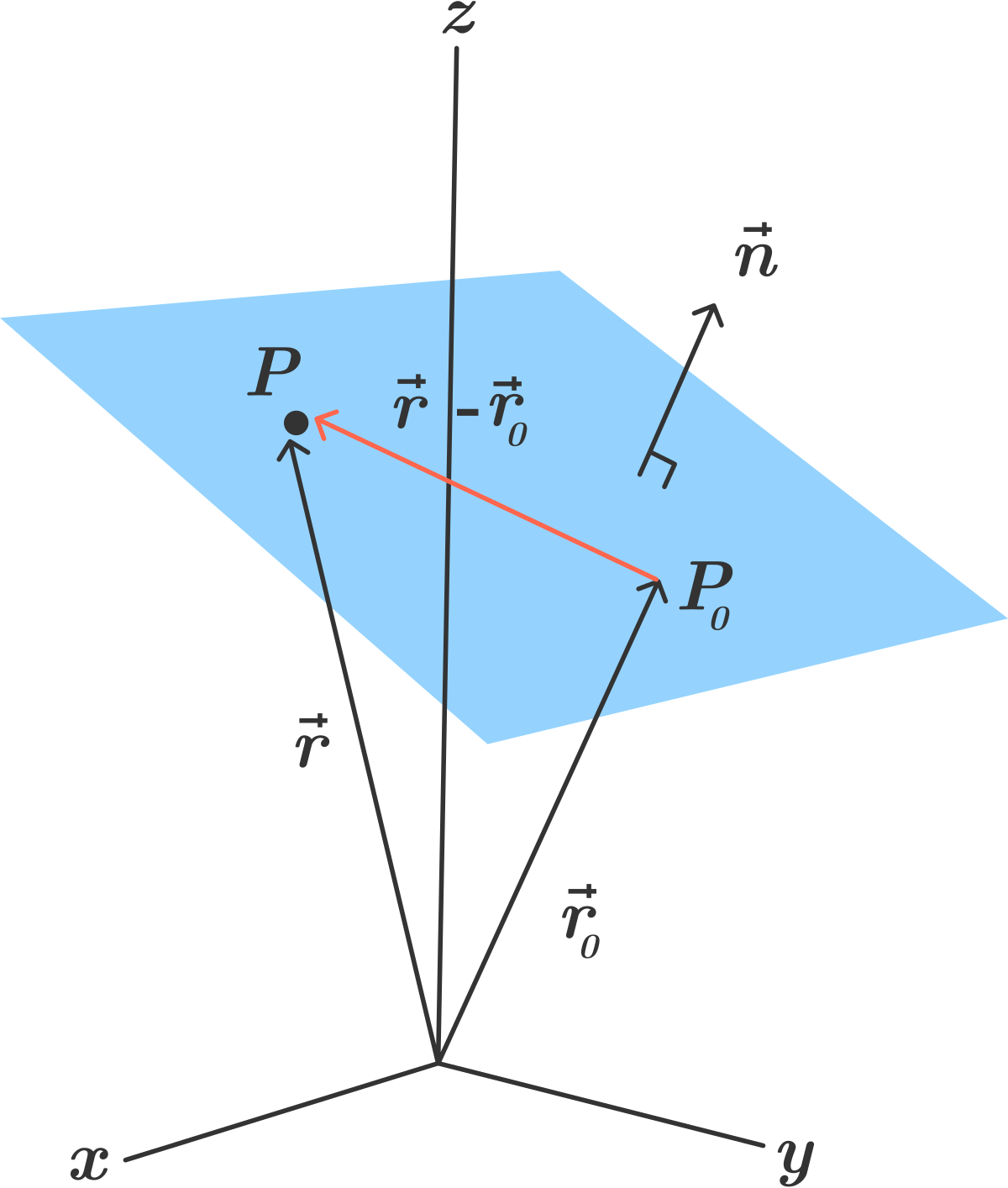


3d Coordinate Geometry Equation Of A Plane Brilliant Math Science Wiki


S0 3



Level Curves Of Functions Of Two Variables Youtube
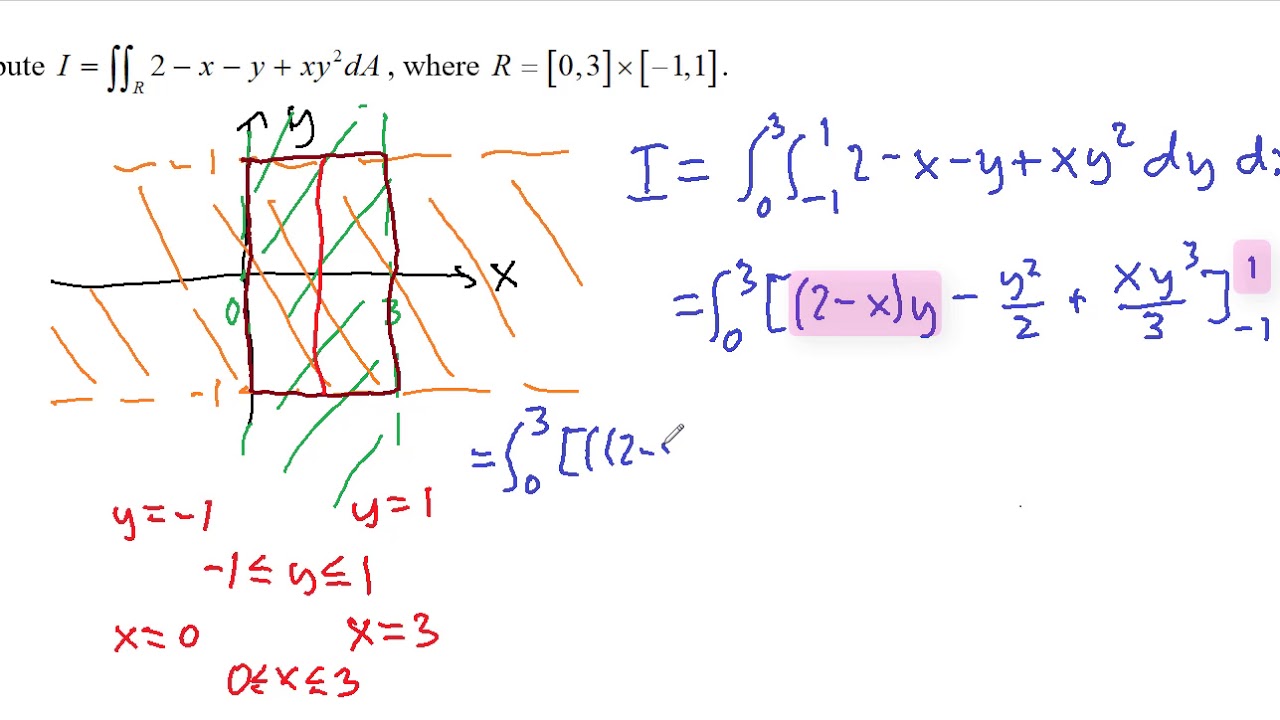


Double Integrals Volume And Average Value



Koval S 3d Grapher
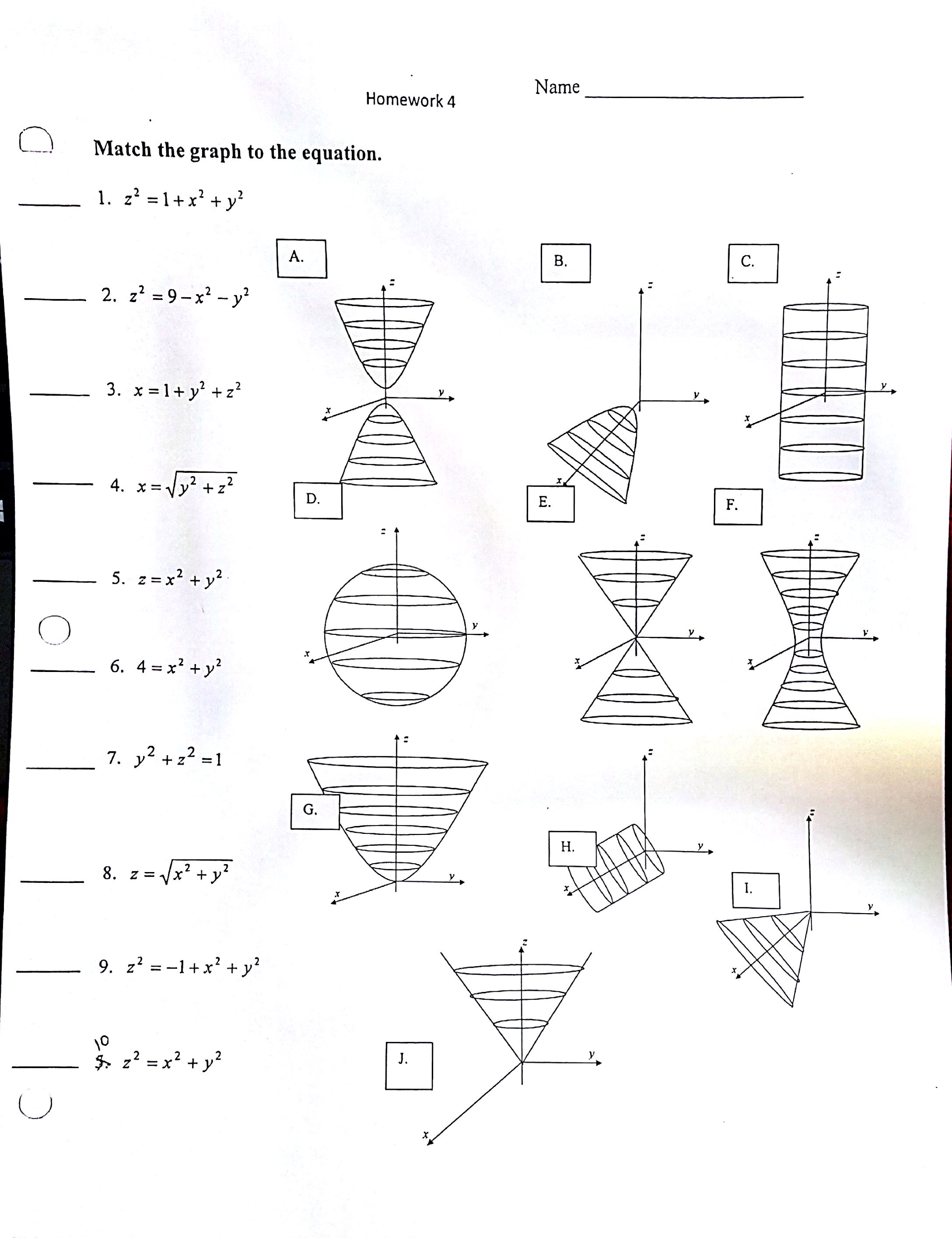


Solved Match The Graph To The Equation X 2 1 X 2 Y Chegg Com
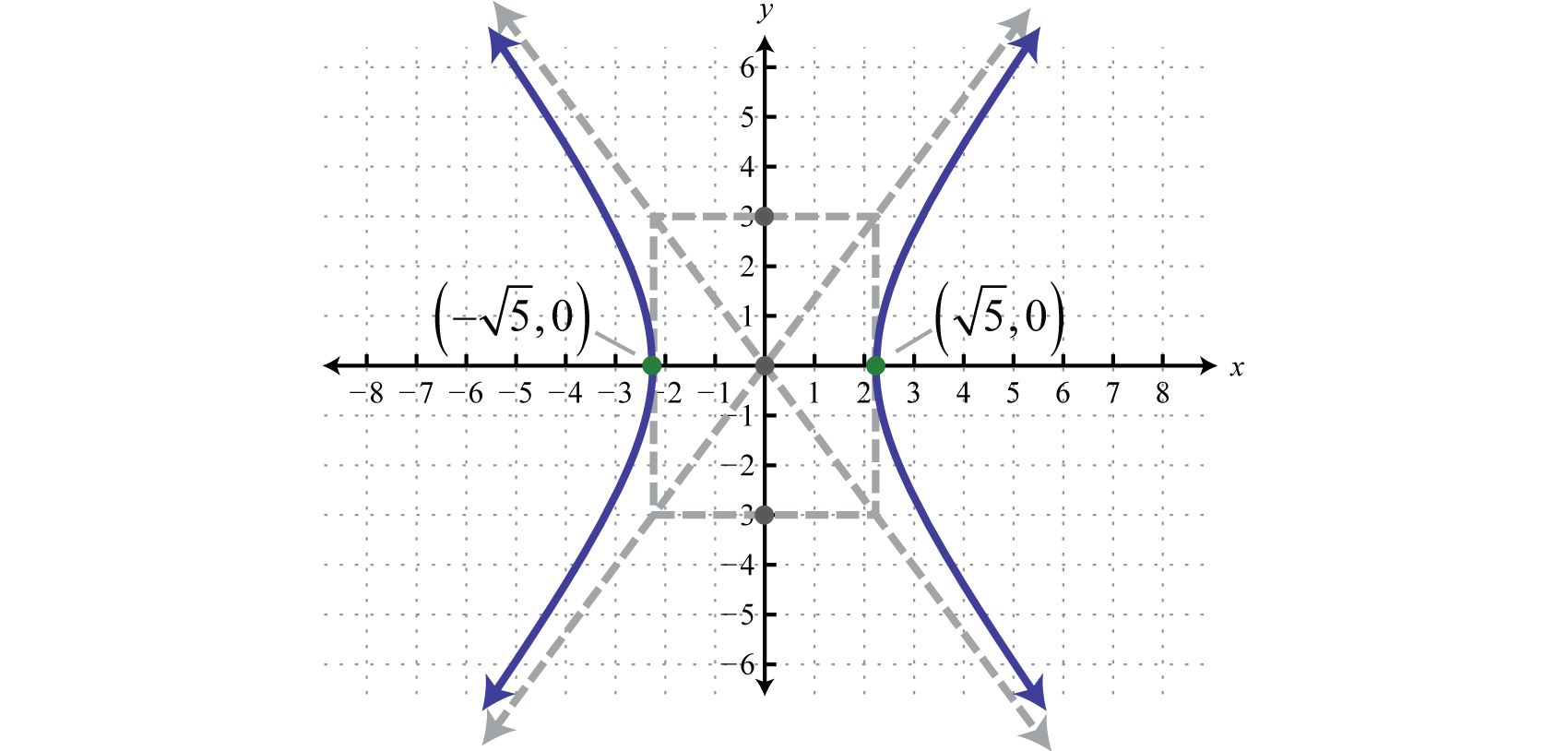


Hyperbolas
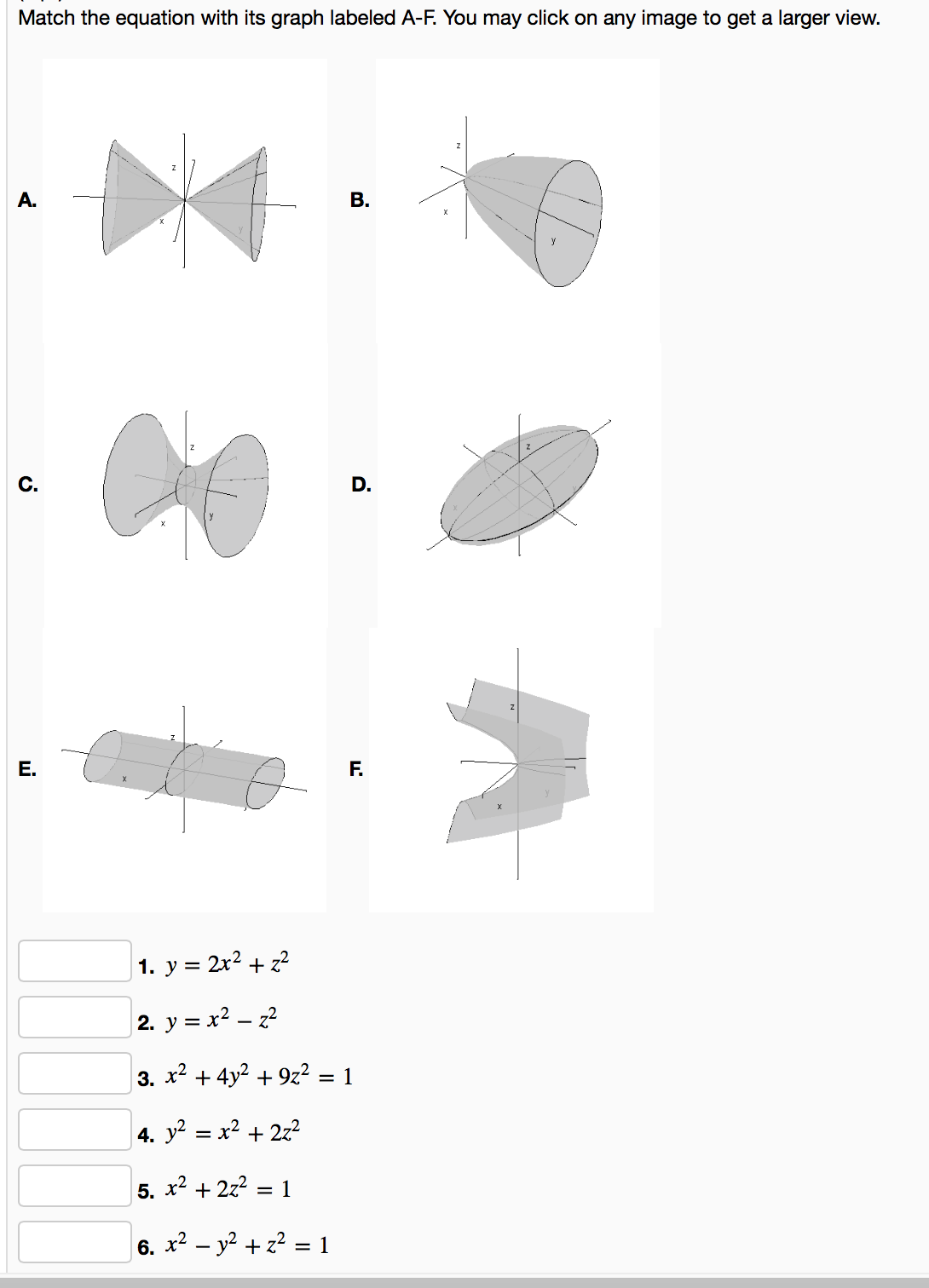


Solved Match The Equation With Its Graph Labeled A F You Chegg Com



Help Choose The Graph That Matches The Following System Of Equations 4x 2y 2 X 3y 24 Brainly Com



0 件のコメント:
コメントを投稿